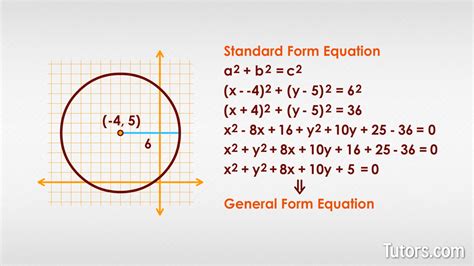
Learning to write the equation of a circle in standard form is an essential skill for anyone studying mathematics, particularly in the fields of geometry and trigonometry. The standard form of a circle's equation is a powerful tool for understanding the properties and behavior of circles. In this article, we will break down the process of writing a circle equation in standard form into five manageable steps.
Understanding the Standard Form
Before we dive into the steps, it's crucial to understand what the standard form of a circle equation looks like. The standard form is given by:
(x - h)^2 + (y - k)^2 = r^2
where (h, k) is the center of the circle, and r is the radius.
Step 1: Identify the Center of the Circle
The first step in writing the equation of a circle in standard form is to identify the center of the circle. The center is the point from which the radius is measured. If you're given the coordinates of the center, you can simply substitute these values into the equation as (h, k). If not, you'll need to find the center using other information, such as the midpoint of a diameter or the intersection of two perpendicular bisectors.

Step 2: Find the Radius of the Circle
Once you have the center, the next step is to find the radius of the circle. The radius is the distance from the center to any point on the circle's circumference. You can use the distance formula or the Pythagorean theorem to find the radius. If you're given the diameter, simply divide by 2 to get the radius.
Step 3: Write the Equation in Standard Form
Now that you have the center (h, k) and radius (r), you can substitute these values into the standard form equation:
(x - h)^2 + (y - k)^2 = r^2
Make sure to plug in the values correctly, paying attention to the signs and parentheses.

Step 4: Simplify the Equation (Optional)
If necessary, simplify the equation by expanding the squared terms and combining like terms. This can help you identify any additional properties of the circle, such as its axis of symmetry.
Step 5: Verify the Equation
Finally, verify that your equation is correct by plugging in a point on the circle's circumference or using graphing software to visualize the circle. This step ensures that your equation accurately represents the circle.

Real-World Applications
Writing the equation of a circle in standard form has numerous real-world applications. For instance, in architecture, circles are used in the design of arches, bridges, and domes. In physics, circular motion is used to describe the orbits of planets and the trajectories of projectiles.

Key Takeaways
- Identify the center (h, k) of the circle.
- Find the radius (r) of the circle.
- Write the equation in standard form: (x - h)^2 + (y - k)^2 = r^2.
- Simplify the equation (if necessary).
- Verify the equation.
By following these five steps, you'll be well on your way to writing the equation of a circle in standard form. Remember to practice regularly to reinforce your understanding of this essential mathematical concept.

What is the standard form of a circle equation?
+The standard form of a circle equation is (x - h)^2 + (y - k)^2 = r^2, where (h, k) is the center and r is the radius.
How do I find the center of a circle?
+You can find the center of a circle using the midpoint of a diameter or the intersection of two perpendicular bisectors.
What is the radius of a circle?
+The radius of a circle is the distance from the center to any point on the circle's circumference.
We hope you found this article helpful in understanding how to write a circle equation in standard form. If you have any questions or comments, please don't hesitate to share them with us.