Polar Form Of 1 I Explained In 5 Minutes

Quick Links :

Unlocking the Secrets of Polar Form: A 5-Minute Guide to Understanding Complex Numbers

Have you ever struggled to understand complex numbers and their representation in polar form? Do you find yourself getting lost in the world of trigonometry and imaginary numbers? Fear not, dear reader, for we are about to embark on a 5-minute journey to unlock the secrets of polar form. By the end of this article, you will have a solid grasp of the concept and be able to tackle even the most daunting complex number problems.

What is Polar Form?

Polar form is a way of representing complex numbers in terms of their magnitude (or length) and direction (or angle). This is in contrast to the more familiar rectangular form, where complex numbers are represented as a sum of real and imaginary parts. The polar form is particularly useful in many areas of mathematics, engineering, and physics, as it allows for easier manipulation and visualization of complex numbers.

How to Convert to Polar Form

Converting a complex number from rectangular form to polar form is a relatively straightforward process. The key is to use the following formulas:

• Magnitude (r) = √(a^2 + b^2)
• Angle (θ) = arctan(b/a)

where a and b are the real and imaginary parts of the complex number, respectively.

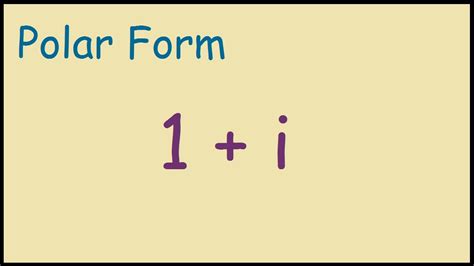
Example: Converting 1 + i to Polar Form

Let's take the complex number 1 + i as an example. Using the formulas above, we can calculate the magnitude and angle as follows:

• Magnitude (r) = √(1^2 + 1^2) = √2
• Angle (θ) = arctan(1/1) = π/4

So, the polar form of 1 + i is √2 ∠ π/4.

Understanding the Polar Form of 1 i

Now that we have a basic understanding of polar form, let's take a closer look at the specific case of 1 i. The polar form of 1 i is simply ∠ π/4, since the magnitude is equal to 1.

But what does this mean in terms of the complex plane? The answer lies in the geometry of the situation. The complex number 1 i corresponds to a point on the unit circle, which is at an angle of π/4 from the positive real axis.

Practical Applications of Polar Form

So, why is polar form important? The answer lies in its many practical applications. Here are just a few examples:

• Electrical Engineering: Polar form is used to analyze and design electrical circuits, particularly those involving AC signals.
• Signal Processing: Polar form is used in signal processing techniques such as filtering and modulation analysis.
• Navigation: Polar form is used in navigation systems, such as GPS, to calculate distances and angles between locations.

Benefits of Using Polar Form

So, what are the benefits of using polar form? Here are just a few:

• Simplifies Complex Number Arithmetic: Polar form makes it easier to perform arithmetic operations on complex numbers, such as multiplication and division.
• Provides Geometric Insight: Polar form provides a geometric interpretation of complex numbers, which can be useful in understanding and visualizing complex number operations.
• Facilitates Trigonometric Analysis: Polar form is particularly useful in trigonometric analysis, as it allows for easier manipulation of angles and magnitudes.

Common Mistakes to Avoid

When working with polar form, there are several common mistakes to avoid:

• Forgetting to Convert to Polar Form: Make sure to convert complex numbers to polar form before performing arithmetic operations or trigonometric analysis.
• Incorrectly Calculating Magnitude and Angle: Double-check your calculations for magnitude and angle to avoid errors.

Conclusion: Mastering Polar Form in 5 Minutes

And there you have it – a comprehensive guide to understanding polar form in just 5 minutes. By mastering polar form, you will be able to tackle even the most complex number problems with confidence. Remember to convert complex numbers to polar form, use the correct formulas for magnitude and angle, and avoid common mistakes.

Now it's your turn! Share your thoughts on polar form in the comments below. Do you have any questions or topics you'd like to discuss? We'd love to hear from you.