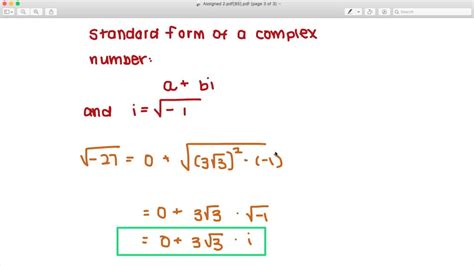
Complex Numbers In Standard Form Made Easy

Quick Links :

Complex numbers are a fundamental concept in mathematics, particularly in algebra and calculus. They are used to extend the real number system to include imaginary numbers, which are essential in solving equations that cannot be expressed on the real number line. One of the most common ways to represent complex numbers is in standard form, which provides a clear and concise way to express these numbers.

What are Complex Numbers in Standard Form?

Complex numbers in standard form are expressed as a + bi, where:

• a is the real part of the complex number
• b is the imaginary part of the complex number
• i is the imaginary unit, which is defined as the square root of -1

For example, the complex number 3 + 4i is in standard form, where 3 is the real part and 4i is the imaginary part.

Why is Standard Form Important?

Standard form is important because it provides a consistent way to express complex numbers, making it easier to perform operations such as addition, subtraction, multiplication, and division. It also allows for easy identification of the real and imaginary parts of a complex number, which is crucial in solving equations and representing complex numbers on the complex plane.

How to Convert Complex Numbers to Standard Form

Converting complex numbers to standard form involves simplifying the expression and separating the real and imaginary parts. Here are the steps:

1. Simplify the expression by combining like terms.
2. Separate the real and imaginary parts by grouping the terms with i together.
3. Write the complex number in standard form, a + bi.

For example, to convert the complex number 2 + 3i + 4 - 2i to standard form, we would simplify the expression by combining like terms:

2 + 3i + 4 - 2i = 6 + i

So, the complex number in standard form is 6 + i.

Converting Complex Numbers with Fractions

When converting complex numbers with fractions, we need to simplify the fraction and separate the real and imaginary parts. Here are the steps:

1. Simplify the fraction by dividing the numerator and denominator by their greatest common divisor.
2. Separate the real and imaginary parts by grouping the terms with i together.
3. Write the complex number in standard form, a + bi.

For example, to convert the complex number 1/2 + 3/4i to standard form, we would simplify the fraction:

1/2 + 3/4i = 2/4 + 3/4i = 5/4 + 3/4i

So, the complex number in standard form is 5/4 + 3/4i.

Operations with Complex Numbers in Standard Form

Performing operations with complex numbers in standard form involves using the rules of algebra and the properties of i. Here are some examples:

• Addition: (a + bi) + (c + di) = (a + c) + (b + d)i
• Subtraction: (a + bi) - (c + di) = (a - c) + (b - d)i
• Multiplication: (a + bi)(c + di) = (ac - bd) + (ad + bc)i
• Division: (a + bi)/(c + di) = ((ac + bd)/(c^2 + d^2)) + ((bc - ad)/(c^2 + d^2))i

For example, to add the complex numbers 2 + 3i and 4 + 2i, we would use the addition rule:

(2 + 3i) + (4 + 2i) = (2 + 4) + (3 + 2)i = 6 + 5i

So, the result is 6 + 5i.

Real-World Applications of Complex Numbers in Standard Form

Complex numbers in standard form have many real-world applications, including:

• Electrical engineering: Complex numbers are used to represent AC circuits and filters.
• Signal processing: Complex numbers are used to represent signals and filters in signal processing.
• Navigation: Complex numbers are used to represent positions and directions in navigation.
• Medical imaging: Complex numbers are used to represent medical images in MRI and CT scans.

For example, in electrical engineering, complex numbers are used to represent AC circuits and filters. The impedance of a circuit is represented as a complex number in standard form, which allows engineers to analyze and design circuits more easily.

Conclusion

In conclusion, complex numbers in standard form are a powerful tool for representing and analyzing complex numbers. By converting complex numbers to standard form, we can simplify expressions, perform operations, and represent complex numbers on the complex plane. The importance of standard form lies in its ability to provide a consistent way to express complex numbers, making it easier to perform operations and solve equations.

We hope this article has helped you understand the concept of complex numbers in standard form. Share your thoughts and comments below!