Ratios and proportions are fundamental concepts in mathematics, and understanding them is crucial for problem-solving in various fields, including science, engineering, and finance. In this article, we will delve into the world of ratios and proportions, exploring what they are, how they work, and their practical applications.
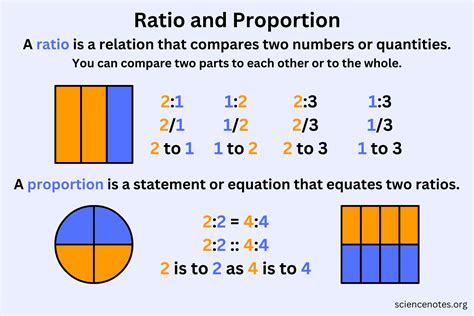
A ratio is a way of comparing two quantities by division, and it can be expressed as a fraction, decimal, or percentage. For instance, the ratio of boys to girls in a class can be written as 3:4, 3/4, or 0.75. Ratios are used to describe relationships between quantities, such as the ratio of the length of a room to its width.
On the other hand, a proportion is a statement that two ratios are equal. It is often represented by the symbol "::" or "=". For example, the proportion 2:3 :: 4:6 states that the ratio of 2 to 3 is equal to the ratio of 4 to 6. Proportions are used to describe equivalent relationships between ratios.
Understanding the Concept of Proportion

To understand proportions, let's consider a simple example. Suppose we have two sets of numbers: 2, 3 and 4, 6. We can write the proportion 2:3 :: 4:6, which means that the ratio of 2 to 3 is equal to the ratio of 4 to 6. To check if this proportion is true, we can convert the ratios to fractions and compare them.
2/3 = 4/6 = 0.67
As we can see, the two ratios are indeed equal, which means the proportion is true.
How to Write a Proportion
Writing a proportion is a straightforward process. We simply write two ratios, separated by the symbol "::" or "=". For example:
2:3 :: 4:6
We can also write proportions using fractions:
2/3 = 4/6
It's essential to remember that when writing proportions, the ratios must be equal. If the ratios are not equal, the proportion is false.
Types of Proportions

There are several types of proportions, including:
- Direct proportion: A direct proportion is a proportion where the two ratios are equal. For example, 2:3 :: 4:6.
- Inverse proportion: An inverse proportion is a proportion where the two ratios are inversely related. For example, 2:3 :: 6:4.
- Part-to-part proportion: A part-to-part proportion is a proportion where one part of a whole is compared to another part. For example, 2 boys: 3 girls :: 4 boys: 6 girls.
- Part-to-whole proportion: A part-to-whole proportion is a proportion where one part of a whole is compared to the entire whole. For example, 2 boys: 5 children :: 4 boys: 10 children.
Real-World Applications of Proportions
Proportions have numerous real-world applications, including:
- Science: Proportions are used in scientific measurements, such as converting between units of measurement.
- Finance: Proportions are used in finance to calculate interest rates, investment returns, and risk management.
- Engineering: Proportions are used in engineering to design and optimize systems, such as bridges and buildings.
- Cooking: Proportions are used in cooking to scale recipes up or down.
Solving Proportions

Solving proportions involves finding the missing value in a proportion. There are several methods to solve proportions, including:
- Cross-multiplication: Cross-multiplication involves multiplying the means and extremes of the proportion to find the missing value.
- Converting to fractions: Converting the proportion to fractions and comparing them can also help solve the proportion.
- Using proportions to solve equations: Proportions can be used to solve equations by setting up a proportion and solving for the unknown variable.
Practical Examples of Solving Proportions
Let's consider a few practical examples of solving proportions:
- Example 1: If 2 boys can do a job in 3 hours, how many boys are needed to do the same job in 4 hours?
- Example 2: If a recipe for 4 people requires 2 cups of flour, how much flour is needed for 6 people?
- Example 3: If a car travels 200 miles in 4 hours, how many miles will it travel in 6 hours?
These examples illustrate the practical applications of proportions and how they can be used to solve real-world problems.
Conclusion
In conclusion, proportions are a fundamental concept in mathematics that have numerous real-world applications. Understanding proportions involves understanding ratios and how to write and solve proportions. With practice and patience, anyone can become proficient in solving proportions and applying them to real-world problems.
We hope this article has helped you understand the concept of proportions and how to solve them. If you have any questions or need further clarification, please don't hesitate to ask.
What is a proportion?
+A proportion is a statement that two ratios are equal.
How do you write a proportion?
+A proportion can be written using the symbol "::" or "=" between two ratios.
What are some real-world applications of proportions?
+Proportions have numerous real-world applications, including science, finance, engineering, and cooking.