Right triangles have been a cornerstone of mathematics and physics for centuries. These triangles, characterized by one right angle (90 degrees), have numerous applications in fields like geometry, trigonometry, and engineering. One of the most interesting aspects of right triangles is the relationship between their side lengths, which can be expressed in various forms and patterns.
Understanding Right Triangles

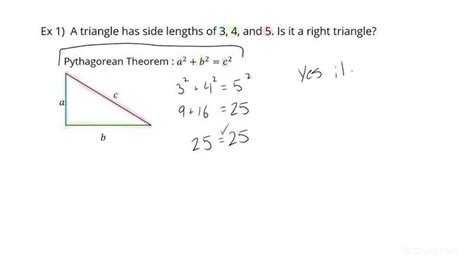
To grasp the concept of right triangles, it's essential to understand the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This theorem is often expressed as a² + b² = c², where a and b are the lengths of the legs, and c is the length of the hypotenuse.
The Importance of Side Lengths in Right Triangles

The side lengths of right triangles play a crucial role in various mathematical and real-world applications. For instance, in construction, knowing the side lengths of a right triangle can help determine the height of a building or the length of a shadow. In physics, right triangles are used to calculate distances, velocities, and forces in problems involving projectile motion or simple harmonic motion.
5 Sets of Side Lengths for Right Triangles
Here are five sets of side lengths for right triangles, each with unique characteristics and applications:
-
3-4-5 Triangle
- Legs: 3, 4
- Hypotenuse: 5
- This triangle is a classic example of a Pythagorean triple, where the ratio of the side lengths is a simple whole number.
-
5-12-13 Triangle
- Legs: 5, 12
- Hypotenuse: 13
- Another Pythagorean triple, this triangle has a wider range of applications, including problems involving larger distances and velocities.
-
7-24-25 Triangle
- Legs: 7, 24
- Hypotenuse: 25
- This triangle is often used in problems involving more complex calculations, such as finding the height of a triangle or the length of a diagonal.
-
8-15-17 Triangle
- Legs: 8, 15
- Hypotenuse: 17
- This triangle is a popular example in geometry and trigonometry, often used to illustrate concepts like sine, cosine, and tangent.
-
9-40-41 Triangle
- Legs: 9, 40
- Hypotenuse: 41
- This triangle has a larger range of side lengths, making it suitable for problems involving bigger scales, such as construction or engineering projects.
Practical Applications of Right Triangles

Right triangles have numerous practical applications in various fields, including:
- Construction: Right triangles are used to calculate the height of buildings, the length of shadows, and the distance between objects.
- Physics: Right triangles are used to calculate distances, velocities, and forces in problems involving projectile motion or simple harmonic motion.
- Engineering: Right triangles are used to design bridges, buildings, and other structures, ensuring stability and balance.
- Navigation: Right triangles are used in navigation systems, such as GPS, to calculate distances and directions.
Benefits of Understanding Right Triangles
Understanding right triangles and their side lengths can bring numerous benefits, including:
- Improved problem-solving skills: By mastering right triangles, you can develop your problem-solving skills and approach complex problems with confidence.
- Enhanced spatial awareness: Right triangles can help you visualize and understand spatial relationships, making you more aware of your surroundings.
- Increased precision: By using right triangles to calculate distances and angles, you can achieve greater precision and accuracy in your work or projects.
Conclusion
Right triangles are a fundamental concept in mathematics and physics, with numerous applications in various fields. By understanding the side lengths of right triangles, you can develop your problem-solving skills, enhance your spatial awareness, and increase your precision. Whether you're a student, a professional, or simply someone interested in mathematics, mastering right triangles can bring numerous benefits and open doors to new possibilities.

If you have any questions or comments about right triangles or their applications, please feel free to share them below. We'd love to hear from you!
What is the Pythagorean theorem?
+The Pythagorean theorem is a mathematical concept that states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. It is often expressed as a² + b² = c².
What are some practical applications of right triangles?
+Right triangles have numerous practical applications in various fields, including construction, physics, engineering, and navigation.
How can I improve my problem-solving skills using right triangles?
+By mastering right triangles and their side lengths, you can develop your problem-solving skills and approach complex problems with confidence.