Right Triangle Side Lengths: What Combinations Work

Quick Links :

Right triangles are a fundamental concept in geometry and trigonometry, and understanding their side lengths is crucial for various mathematical and real-world applications. In this article, we will delve into the world of right triangle side lengths, exploring what combinations work and why.

Right triangles are characterized by one right angle (90 degrees) and two acute angles (less than 90 degrees). The side opposite the right angle is called the hypotenuse, and the other two sides are referred to as legs. The relationships between these side lengths are governed by the Pythagorean theorem, which states that the square of the hypotenuse is equal to the sum of the squares of the legs.

Understanding the Pythagorean Theorem

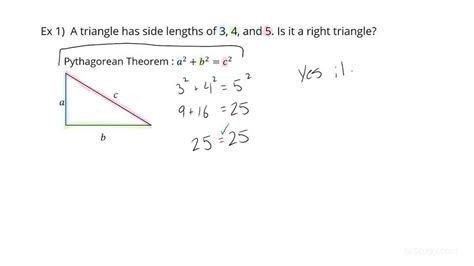
The Pythagorean theorem is a fundamental concept in geometry, and it is essential for understanding right triangle side lengths. The theorem states that:

a^2 + b^2 = c^2

where a and b are the lengths of the legs, and c is the length of the hypotenuse.

This theorem can be used to find the length of the hypotenuse, given the lengths of the legs, or to find the lengths of the legs, given the length of the hypotenuse.

Pythagorean Triples

Pythagorean triples are sets of three positive integers that satisfy the Pythagorean theorem. These triples can be used to generate right triangles with integer side lengths. For example:

• 3-4-5: This is one of the most well-known Pythagorean triples, where 3 and 4 are the lengths of the legs, and 5 is the length of the hypotenuse.
• 5-12-13: This is another Pythagorean triple, where 5 and 12 are the lengths of the legs, and 13 is the length of the hypotenuse.

Pythagorean triples can be used to create right triangles with integer side lengths, making them useful for various mathematical and real-world applications.

Combinations of Right Triangle Side Lengths

Now that we have a basic understanding of the Pythagorean theorem and Pythagorean triples, let's explore some combinations of right triangle side lengths that work.

• Integer side lengths: As we discussed earlier, Pythagorean triples can be used to generate right triangles with integer side lengths.
• Rational side lengths: Rational numbers can also be used to create right triangles with rational side lengths. For example, 3/4 and 4/5 can be used to create a right triangle with rational side lengths.
• Irrational side lengths: Irrational numbers, such as the square root of 2, can also be used to create right triangles with irrational side lengths.

Special Right Triangles

There are several special right triangles that have unique properties and are used frequently in mathematics and real-world applications. Some examples include:

• 45-45-90 triangles: These triangles have two equal legs and a hypotenuse that is √2 times the length of each leg.
• 30-60-90 triangles: These triangles have one leg that is half the length of the hypotenuse, and the other leg is √3 times the length of the first leg.

These special right triangles are used frequently in trigonometry and geometry, and are essential for solving various mathematical and real-world problems.

Real-World Applications of Right Triangle Side Lengths

Right triangle side lengths have numerous real-world applications in fields such as physics, engineering, and architecture. Some examples include:

• Building design: Right triangles are used to design buildings and bridges, ensuring that they are structurally sound and aesthetically pleasing.
• Physics and engineering: Right triangles are used to calculate distances, velocities, and forces in physics and engineering applications.
• GPS technology: Right triangles are used in GPS technology to calculate distances and locations.

Conclusion

In conclusion, right triangle side lengths are a fundamental concept in geometry and trigonometry, and understanding their combinations is essential for various mathematical and real-world applications. From Pythagorean triples to special right triangles, there are numerous combinations of right triangle side lengths that work, and each has its unique properties and applications.

We hope this article has provided you with a comprehensive understanding of right triangle side lengths and their combinations. If you have any questions or comments, please feel free to share them with us.