Unlocking the Power of Trigonometric Form

In the realm of complex numbers, there exists a powerful tool that can simplify even the most daunting expressions: the trigonometric form. This form, also known as polar form, allows us to represent complex numbers in a way that is both intuitive and convenient. By leveraging the principles of trigonometry, we can unlock the secrets of complex numbers and make calculations a breeze.
The Need for Trigonometric Form
When working with complex numbers, it's not uncommon to encounter expressions that seem impossible to simplify. The standard form of complex numbers, which represents them as a + bi, can be cumbersome to work with, especially when dealing with multiplication and division. This is where the trigonometric form comes in – a game-changing alternative that can simplify complex calculations and reveal new insights.
What is Trigonometric Form?

The trigonometric form of a complex number is a way of representing it using trigonometric functions, specifically the sine and cosine functions. In this form, a complex number is expressed as:
z = r(cosθ + isinθ)
where:
- z is the complex number
- r is the magnitude (or length) of the complex number
- θ is the angle formed by the complex number with the positive x-axis
This form may seem unfamiliar at first, but it's actually a natural extension of the way we represent points in the Cartesian plane.
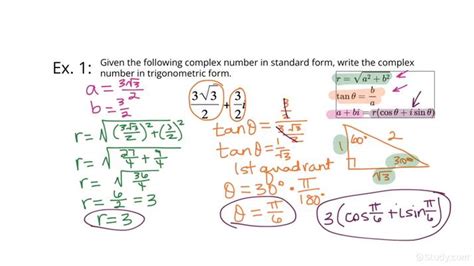
How to Convert to Trigonometric Form
Converting a complex number to its trigonometric form is relatively straightforward. Here are the steps:
- Find the magnitude (r) of the complex number using the formula: r = √(a^2 + b^2)
- Find the angle (θ) using the formula: θ = arctan(b/a)
- Express the complex number in trigonometric form using the formula: z = r(cosθ + isinθ)
For example, let's convert the complex number 3 + 4i to its trigonometric form.
First, we find the magnitude: r = √(3^2 + 4^2) = 5
Next, we find the angle: θ = arctan(4/3) = 53.13°
Finally, we express the complex number in trigonometric form: z = 5(cos53.13° + isin53.13°)
Benefits of Trigonometric Form

The trigonometric form offers several benefits that make it a powerful tool for working with complex numbers.
- Simplifies calculations: Trigonometric form makes it easy to perform calculations such as multiplication and division.
- Reveals geometric insights: By representing complex numbers as points in the Cartesian plane, trigonometric form provides a visual understanding of complex number arithmetic.
- Eases conversion between forms: Trigonometric form makes it easy to convert between different forms of complex numbers, such as rectangular and polar form.
Practical Applications of Trigonometric Form
Trigonometric form has numerous practical applications in fields such as:
- Electrical engineering: Trigonometric form is used to analyze and design AC circuits.
- Signal processing: Trigonometric form is used to represent and manipulate signals in signal processing.
- Navigation: Trigonometric form is used in navigation systems to represent positions and velocities.
Common Operations in Trigonometric Form

When working with complex numbers in trigonometric form, it's essential to understand how to perform common operations such as addition, subtraction, multiplication, and division.
- Addition and subtraction: To add or subtract complex numbers in trigonometric form, simply add or subtract the corresponding magnitudes and angles.
- Multiplication and division: To multiply or divide complex numbers in trigonometric form, multiply or divide the corresponding magnitudes and add or subtract the corresponding angles.
For example, let's multiply two complex numbers in trigonometric form: z1 = 3(cos30° + isin30°) and z2 = 2(cos45° + isin45°).
First, we multiply the magnitudes: r1r2 = 3 × 2 = 6
Next, we add the angles: θ1 + θ2 = 30° + 45° = 75°
Finally, we express the result in trigonometric form: z1z2 = 6(cos75° + isin75°)
De Moivre's Theorem

De Moivre's theorem is a fundamental result in trigonometry that allows us to raise complex numbers to integer powers. The theorem states that:
(cosθ + isinθ)^n = cos(nθ) + isin(nθ)
This theorem has numerous applications in mathematics and engineering, particularly in the analysis of periodic phenomena.
Conclusion: Unlocking the Power of Trigonometric Form
The trigonometric form is a powerful tool for working with complex numbers. By representing complex numbers in a way that is both intuitive and convenient, trigonometric form simplifies calculations, reveals geometric insights, and eases conversion between forms. Whether you're a student, teacher, or professional, mastering the trigonometric form will unlock new possibilities for working with complex numbers.
What is the main benefit of using trigonometric form?
+The main benefit of using trigonometric form is that it simplifies calculations and provides a visual understanding of complex number arithmetic.
How do I convert a complex number to its trigonometric form?
+To convert a complex number to its trigonometric form, find the magnitude (r) and angle (θ) using the formulas: r = √(a^2 + b^2) and θ = arctan(b/a). Then, express the complex number in trigonometric form using the formula: z = r(cosθ + isinθ).
What is De Moivre's theorem?
+De Moivre's theorem is a fundamental result in trigonometry that allows us to raise complex numbers to integer powers. The theorem states that: (cosθ + isinθ)^n = cos(nθ) + isin(nθ).