The ellipse is one of the most fundamental concepts in mathematics, particularly in geometry and algebra. It is a closed curve on a plane surrounding two focal points such that the sum of the distances to the two focal points is constant. The ellipse has numerous applications in various fields, including astronomy, engineering, and art. In this article, we will delve into the standard form equation of the ellipse, exploring its components, benefits, and practical applications.
What is an Ellipse?

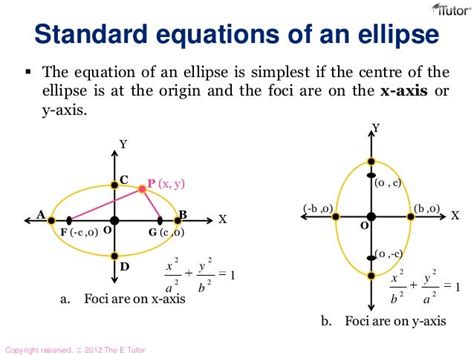
An ellipse is a type of curve that is symmetrical about two axes, known as the major and minor axes. The major axis is the longest diameter of the ellipse, while the minor axis is the shortest diameter. The ellipse has two focal points, which are located on the major axis and are equidistant from the center of the ellipse. The sum of the distances from any point on the ellipse to the two focal points is constant.
Standard Form Equation of the Ellipse

The standard form equation of the ellipse is:
(x - h)^2/a^2 + (y - k)^2/b^2 = 1
where:
- (h, k) is the center of the ellipse
- a is the semi-major axis (the distance from the center to the farthest point on the ellipse)
- b is the semi-minor axis (the distance from the center to the closest point on the ellipse)
This equation represents the general form of an ellipse, which can be modified to fit specific types of ellipses, such as circles and ellipses with different orientations.
Components of the Standard Form Equation
The standard form equation of the ellipse consists of several components that work together to define the shape and size of the ellipse.
- The center (h, k) determines the position of the ellipse on the coordinate plane.
- The semi-major axis (a) determines the length of the major axis, which is the longest diameter of the ellipse.
- The semi-minor axis (b) determines the length of the minor axis, which is the shortest diameter of the ellipse.
Benefits of the Standard Form Equation

The standard form equation of the ellipse has several benefits that make it a powerful tool in mathematics and real-world applications.
- Universality: The standard form equation can be used to represent any type of ellipse, regardless of its size, shape, or orientation.
- Flexibility: The equation can be modified to fit specific types of ellipses, such as circles and ellipses with different orientations.
- Simplifies Calculations: The standard form equation simplifies calculations involving ellipses, making it easier to perform tasks such as finding the area, perimeter, and eccentricity of the ellipse.
Practical Applications of the Standard Form Equation

The standard form equation of the ellipse has numerous practical applications in various fields, including:
- Astronomy: Ellipses are used to describe the orbits of planets and stars in our solar system and beyond.
- Engineering: Ellipses are used in the design of structures such as bridges, tunnels, and buildings.
- Art: Ellipses are used in the creation of artistic designs, such as logos and graphics.
Steps to Graph an Ellipse Using the Standard Form Equation

To graph an ellipse using the standard form equation, follow these steps:
- Identify the center (h, k) of the ellipse.
- Determine the semi-major axis (a) and semi-minor axis (b) of the ellipse.
- Plot the vertices of the ellipse, which are located at (h ± a, k) and (h, k ± b).
- Draw a smooth curve through the vertices to complete the ellipse.
Conclusion

In conclusion, the standard form equation of the ellipse is a powerful tool that has numerous benefits and practical applications. By understanding the components of the equation and how to graph an ellipse, you can unlock the secrets of this fundamental concept in mathematics. Whether you're a student, teacher, or professional, the standard form equation of the ellipse is an essential tool to have in your toolkit.
We encourage you to share your thoughts and questions about the standard form equation of the ellipse in the comments section below.
What is the difference between a circle and an ellipse?
+A circle is a special type of ellipse where the semi-major axis (a) is equal to the semi-minor axis (b). In other words, a circle is an ellipse with equal axes.
How do I determine the center of an ellipse?
+The center of an ellipse is located at the midpoint of the major axis. To find the center, simply average the x-coordinates of the vertices and the y-coordinates of the vertices.
What is the eccentricity of an ellipse?
+The eccentricity of an ellipse is a measure of how elliptical it is. It is calculated as the ratio of the distance from the center to a focus to the distance from the center to a vertex.