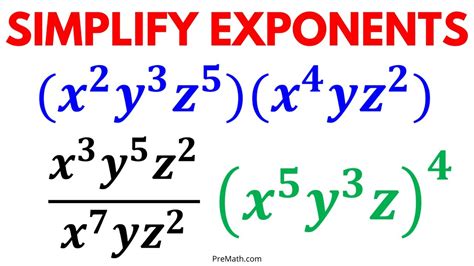Simplifying Exponents: 1 Way To Calculate X^4

Quick Links :

Exponents are a fundamental concept in mathematics, used to represent repeated multiplication of a number by itself. They can be quite daunting, especially when dealing with large values. However, there are ways to simplify exponents, making calculations more manageable. In this article, we will explore one method to calculate x^4, and discuss the importance of exponents in various mathematical operations.

Understanding Exponents

Exponents are used to represent the repeated multiplication of a number by itself. For example, 2^3 represents 2 multiplied by itself 3 times, or 2 × 2 × 2 = 8. Exponents can be positive, negative, or zero, and can be expressed as a fraction or a whole number. The base number, in this case, 2, is raised to the power of the exponent, 3.

Importance of Exponents

Exponents play a crucial role in various mathematical operations, including algebra, geometry, and calculus. They are used to describe the growth and decay of quantities, and to model real-world phenomena, such as population growth, chemical reactions, and electrical circuits. Exponents are also essential in finance, where they are used to calculate interest rates, investment returns, and risk management.

Method to Calculate x^4

One way to calculate x^4 is by using the formula:

x^4 = (x^2)^2

This method involves squaring the value of x twice. For example, if we want to calculate 3^4, we can use the formula:

3^4 = (3^2)^2 = (9)^2 = 81

This method is particularly useful when dealing with large values of x, as it reduces the number of calculations required.

Benefits of this Method

This method has several benefits, including:

• Reduced calculations: By squaring the value of x twice, we reduce the number of calculations required, making it easier to calculate large values of x^4.
• Simplified calculations: This method simplifies the calculation of x^4, making it more manageable and less prone to errors.
• Improved accuracy: By using this method, we can improve the accuracy of our calculations, especially when dealing with large values of x.

Applications of x^4

The calculation of x^4 has several applications in various fields, including:

• Algebra: x^4 is used to solve equations, such as quadratic equations, and to calculate the roots of polynomial equations.
• Geometry: x^4 is used to calculate the area and volume of geometric shapes, such as squares, rectangles, and cubes.
• Physics: x^4 is used to calculate the energy and momentum of particles, and to model the behavior of physical systems.

Real-World Examples

The calculation of x^4 has several real-world examples, including:

• Calculating the area of a square: If we want to calculate the area of a square with a side length of 3 meters, we can use the formula:

Area = x^2 = 3^2 = 9 square meters

• Calculating the volume of a cube: If we want to calculate the volume of a cube with a side length of 4 meters, we can use the formula:

Volume = x^3 = 4^3 = 64 cubic meters

Common Mistakes to Avoid

When calculating x^4, there are several common mistakes to avoid, including:

• Forgetting to square the value of x twice
• Incorrectly applying the formula
• Rounding errors

Tips and Tricks

To avoid common mistakes and improve accuracy, here are some tips and tricks:

• Use a calculator or software to check calculations
• Double-check calculations for accuracy
• Use a consistent method for calculating x^4

Conclusion: Simplifying Exponents

In conclusion, simplifying exponents is an essential skill in mathematics, and the method of calculating x^4 by squaring the value of x twice is a useful technique to master. By understanding the importance of exponents, using the correct formula, and avoiding common mistakes, we can improve our accuracy and confidence in mathematical calculations.

We hope this article has been informative and helpful in your mathematical journey. Do you have any questions or comments about simplifying exponents or calculating x^4? Please share your thoughts in the comments section below.