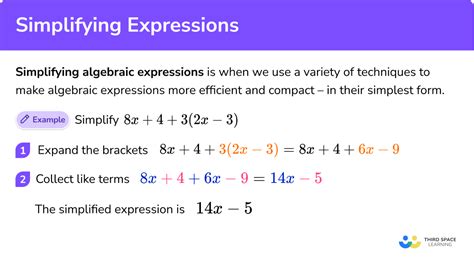
Algebra can be a daunting subject for many students, but with the right approach, it can be made more manageable. One of the fundamental skills in algebra is simplifying expressions, which involves combining like terms and manipulating the equation to make it easier to work with. In this article, we will break down the process of simplifying algebraic expressions into three easy steps.
Step 1: Combine Like Terms

The first step in simplifying an algebraic expression is to combine like terms. Like terms are terms that have the same variable(s) raised to the same power. For example, 2x and 3x are like terms because they both have the variable x raised to the same power (which is 1). To combine like terms, we simply add or subtract their coefficients.
Here are some examples of combining like terms:
- 2x + 3x = 5x
- 4y - 2y = 2y
- 3z + 2z = 5z
What are Coefficients?
Coefficients are the numbers that are multiplied by the variable(s) in an algebraic expression. For example, in the expression 2x, the coefficient is 2. In the expression 3y, the coefficient is 3.
Step 2: Distribute and Combine

Once we have combined like terms, the next step is to distribute and combine any remaining terms. To distribute, we need to multiply a single term or a group of terms by another term or group of terms.
Here are some examples of distributing and combining:
- 2(x + 3) = 2x + 6
- 3(y - 2) = 3y - 6
- 4(z + 2) = 4z + 8
Note that when we distribute, we need to multiply each term inside the parentheses by the term outside the parentheses.
What is the Order of Operations?
The order of operations is a set of rules that tells us which operations to perform first when we have multiple operations in an expression. The order of operations is as follows:
- Parentheses: Evaluate any expressions inside parentheses first.
- Exponents: Evaluate any exponents next.
- Multiplication and Division: Evaluate any multiplication and division operations from left to right.
- Addition and Subtraction: Finally, evaluate any addition and subtraction operations from left to right.
Step 3: Simplify Any Remaining Terms

The final step in simplifying an algebraic expression is to simplify any remaining terms. This may involve combining any remaining like terms or rearranging the expression to make it easier to work with.
Here are some examples of simplifying remaining terms:
- 2x + 5 - 3 = 2x + 2
- 4y - 2 + 1 = 4y - 1
- 3z + 2 - 1 = 3z + 1
Tips and Tricks
Here are some tips and tricks to help you simplify algebraic expressions:
- Always combine like terms first.
- Use the order of operations to ensure that you are performing operations in the correct order.
- Simplify any remaining terms to make the expression easier to work with.
- Practice, practice, practice! Simplifying algebraic expressions takes practice, so be sure to practice simplifying different types of expressions.
What is the difference between a coefficient and a variable?
+A coefficient is a number that is multiplied by a variable, while a variable is a letter or symbol that represents a value that can change.
How do I distribute terms in an algebraic expression?
+To distribute terms, multiply each term inside the parentheses by the term outside the parentheses.
What is the order of operations?
+The order of operations is a set of rules that tells us which operations to perform first when we have multiple operations in an expression. The order of operations is parentheses, exponents, multiplication and division, and addition and subtraction.
In conclusion, simplifying algebraic expressions involves combining like terms, distributing and combining, and simplifying any remaining terms. By following these three easy steps and practicing with different types of expressions, you can become proficient in simplifying algebraic expressions. Remember to use the order of operations and to simplify any remaining terms to make the expression easier to work with.