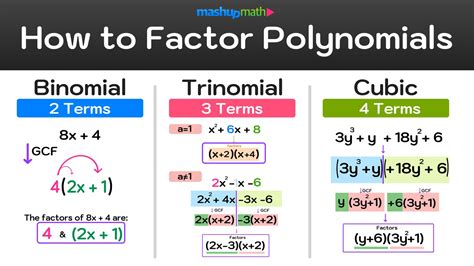
Understanding the Concept of Factored Form
The factored form of a polynomial expression is a fundamental concept in algebra, allowing us to express complex expressions in a simpler and more manageable way. In this article, we will delve into the concept of factored form, specifically focusing on the expression P(4, 16). By breaking down this expression, we aim to provide a comprehensive understanding of the factored form and its significance in mathematical problem-solving.
What is Factored Form?
Factored form is a way of expressing a polynomial expression as a product of its factors. In other words, it involves breaking down a complex expression into simpler components, making it easier to analyze and solve problems. This form is particularly useful when dealing with quadratic equations, as it allows us to easily identify the roots of the equation.
The Expression P(4, 16)

The expression P(4, 16) represents a polynomial equation of the form x^2 - 4x - 16 = 0. Our goal is to factorize this expression, breaking it down into simpler factors that can be easily analyzed.
Factoring the Expression P(4, 16)
To factorize the expression P(4, 16), we need to find two numbers whose product is -16 and whose sum is -4. By analyzing the expression, we can see that the numbers -8 and 2 satisfy these conditions.
P(4, 16) = x^2 - 4x - 16 = (x - 8)(x + 2) = 0
As we can see, the factored form of P(4, 16) is (x - 8)(x + 2) = 0. This tells us that either (x - 8) = 0 or (x + 2) = 0.
Benefits of Factored Form
The factored form of a polynomial expression offers several benefits, including:
• Easier analysis: Factored form makes it easier to analyze the expression, identify its roots, and determine its behavior. • Simplified problem-solving: Factored form simplifies problem-solving, as it allows us to work with simpler components rather than complex expressions. • Improved understanding: Factored form provides a deeper understanding of the underlying structure of the expression, making it easier to identify patterns and relationships.
Applications of Factored Form
Factored form has numerous applications in various fields, including:
• Algebra: Factored form is used to solve quadratic equations, analyze polynomial expressions, and determine the roots of equations. • Calculus: Factored form is used to analyze functions, determine their behavior, and identify maxima and minima. • Physics: Factored form is used to model real-world phenomena, analyze complex systems, and determine the behavior of physical systems.
Conclusion and Next Steps
In this article, we have explored the concept of factored form, specifically focusing on the expression P(4, 16). By breaking down this expression, we have demonstrated the benefits and applications of factored form. As we continue to explore the world of algebra and mathematics, it is essential to develop a deep understanding of factored form and its significance in problem-solving.
Call to Action
We invite you to share your thoughts and experiences with factored form in the comments below. Have you encountered any challenging problems that required the use of factored form? How has factored form helped you in your mathematical journey? Share your stories, and let's continue the conversation!
What is the difference between factored form and expanded form?
+Factored form is a way of expressing a polynomial expression as a product of its factors, while expanded form is a way of expressing a polynomial expression as a sum of its terms.
How do I determine the factors of a polynomial expression?
+To determine the factors of a polynomial expression, look for two numbers whose product is the constant term and whose sum is the coefficient of the linear term.
What are the benefits of using factored form in problem-solving?
+Factored form makes it easier to analyze the expression, identify its roots, and determine its behavior. It also simplifies problem-solving and provides a deeper understanding of the underlying structure of the expression.