Simplifying Radicals: What Is Simplest Radical Form

Quick Links :

Understanding Radicals and Their Importance in Math

Radicals, also known as roots, are an essential part of mathematics, particularly in algebra and geometry. They are used to represent a number that, when multiplied by itself, gives a specified value. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16. In this article, we will delve into the world of radicals, exploring what they are, why they are important, and how to simplify them.

Radicals have been a cornerstone of mathematics for centuries, with applications in various fields, including physics, engineering, and computer science. They are used to solve equations, calculate distances, and model real-world phenomena. Understanding radicals is crucial for anyone interested in pursuing a career in science, technology, engineering, and mathematics (STEM).

The Importance of Simplifying Radicals

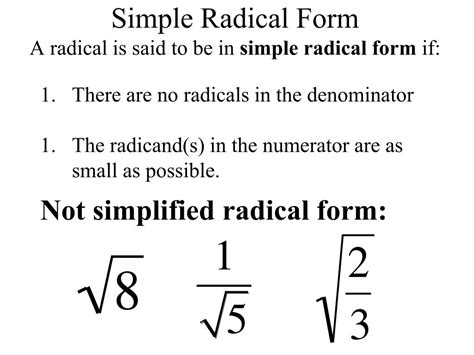
Simplifying radicals is a crucial step in working with these mathematical expressions. A simplified radical is one that is written in its most basic form, with no perfect squares or cubes in the radicand (the number inside the radical). Simplifying radicals helps to:

• Reduce complexity: Simplified radicals make it easier to work with mathematical expressions, reducing the complexity and making it more manageable.
• Improve accuracy: Simplifying radicals ensures that calculations are accurate and reliable, reducing the risk of errors.
• Enhance understanding: Simplified radicals provide a deeper understanding of the underlying mathematical concepts, making it easier to analyze and solve problems.

What Is Simplest Radical Form?

The simplest radical form is a way of writing a radical expression in its most basic form. This involves removing any perfect squares or cubes from the radicand and expressing the radical in terms of the smallest possible integer. For example, the radical expression √16 can be simplified to 4, because 4 multiplied by 4 equals 16.

To simplify a radical expression, follow these steps:

1. Factor the radicand into its prime factors.
2. Identify any perfect squares or cubes in the radicand.
3. Remove the perfect squares or cubes from the radicand.
4. Express the radical in terms of the smallest possible integer.

Types of Radicals

There are several types of radicals, including:

• Square roots: These are the most common type of radical, denoted by the symbol √.
• Cube roots: These are denoted by the symbol ³√.
• Fourth roots: These are denoted by the symbol ⁴√.
• Higher-order roots: These are denoted by the symbol ⁿ√, where n is the order of the root.

Simplifying Radical Expressions

Simplifying radical expressions involves applying the rules of radicals to reduce the complexity of the expression. Here are some examples:

• Simplify √16: √16 = √(4 × 4) = 4
• Simplify ³√27: ³√27 = ³√(3 × 3 × 3) = 3
• Simplify √48: √48 = √(16 × 3) = 4√3

Rules for Simplifying Radicals

When simplifying radicals, follow these rules:

• The product rule: √(ab) = √a × √b
• The quotient rule: √(a/b) = √a / √b
• The power rule: (√a)ⁿ = √(aⁿ)

Real-World Applications of Radicals

Radicals have numerous real-world applications, including:

• Physics: Radicals are used to calculate distances, velocities, and accelerations.
• Engineering: Radicals are used to design and optimize systems, such as bridges and electronic circuits.
• Computer Science: Radicals are used in algorithms for image processing, data compression, and cryptography.

Challenges and Limitations of Radicals

While radicals are powerful mathematical tools, they also have limitations and challenges. Some of the challenges include:

• Complexity: Radical expressions can be complex and difficult to work with.
• Ambiguity: Radical expressions can be ambiguous, with multiple possible interpretations.
• Numerical instability: Radical expressions can be sensitive to numerical errors, leading to instability.

Conclusion

Radicals are an essential part of mathematics, with numerous real-world applications. Simplifying radicals is a crucial step in working with these mathematical expressions, reducing complexity and improving accuracy. By understanding the rules and techniques for simplifying radicals, you can unlock the power of these mathematical tools and apply them to a wide range of problems.

We encourage you to share your thoughts and questions about radicals in the comments section below. How do you use radicals in your everyday life? What challenges have you faced when working with radical expressions?