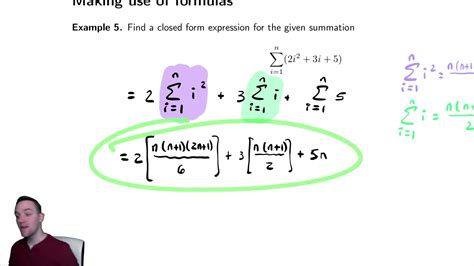
Unlocking the secrets of mathematics can be a daunting task, especially when it comes to dealing with complex expressions. However, with the right tools and techniques, anyone can learn to simplify and manipulate mathematical expressions with ease. In this article, we will explore the concept of closed form expressions and provide five essential keys to help you unlock their secrets.
Mathematics is a language that uses symbols, equations, and formulas to describe the world around us. It is a powerful tool that can be used to model real-world phenomena, make predictions, and optimize solutions. However, mathematical expressions can quickly become complicated and unwieldy, making it difficult to extract meaningful insights. This is where closed form expressions come in – a mathematical expression that can be written in a finite and compact form, often using special functions or mathematical constants.

What are Closed Form Expressions?
Closed form expressions are mathematical expressions that can be written in a finite and compact form, often using special functions or mathematical constants. They are called "closed" because they have a specific and well-defined form, unlike open-ended or infinite series. Closed form expressions are essential in mathematics and science, as they provide a concise and elegant way to describe complex phenomena.
Key 1: Mastering Algebraic Manipulations
The first key to unlocking closed form expressions is to master algebraic manipulations. Algebra is a branch of mathematics that deals with the study of variables and their relationships. It provides a powerful toolkit for manipulating mathematical expressions, including factoring, expanding, and simplifying. By mastering algebraic manipulations, you can simplify complex expressions and rewrite them in a more compact form.
Some essential algebraic manipulations include:
- Factoring: breaking down an expression into its constituent parts
- Expanding: multiplying out an expression to reveal its underlying structure
- Simplifying: combining like terms and eliminating unnecessary complexity
Key 2: Understanding Special Functions
The second key to unlocking closed form expressions is to understand special functions. Special functions are mathematical functions that have specific properties and behaviors, often used to describe real-world phenomena. They include functions like the exponential function, trigonometric functions, and logarithmic functions. By understanding special functions, you can identify and manipulate closed form expressions with ease.
Some essential special functions include:
- Exponential function: a function that grows or decays exponentially
- Trigonometric functions: functions that describe periodic phenomena, like sine and cosine
- Logarithmic function: a function that describes the inverse of exponential growth

Key 3: Working with Mathematical Constants
The third key to unlocking closed form expressions is to work with mathematical constants. Mathematical constants are values that remain unchanged, often used to describe fundamental properties of the universe. They include constants like pi, e, and the golden ratio. By working with mathematical constants, you can identify and manipulate closed form expressions that describe real-world phenomena.
Some essential mathematical constants include:
- Pi: the ratio of a circle's circumference to its diameter
- e: the base of the natural logarithm
- The golden ratio: a value that describes the proportions of many natural phenomena
Key 4: Using Calculus to Simplify Expressions
The fourth key to unlocking closed form expressions is to use calculus to simplify expressions. Calculus is a branch of mathematics that deals with the study of rates of change and accumulation. It provides a powerful toolkit for simplifying complex expressions, including differentiation and integration. By using calculus, you can simplify closed form expressions and reveal their underlying structure.
Some essential calculus techniques include:
- Differentiation: finding the rate of change of a function
- Integration: finding the accumulation of a function

Key 5: Practicing with Real-World Examples
The fifth and final key to unlocking closed form expressions is to practice with real-world examples. By working with real-world examples, you can develop your skills and intuition for identifying and manipulating closed form expressions. Try working with examples from physics, engineering, or economics, and see if you can simplify complex expressions using the techniques outlined above.
Some essential real-world examples include:
- Modeling population growth using exponential functions
- Describing the motion of objects using trigonometric functions
- Optimizing economic systems using calculus

Unlocking the Secrets of Closed Form Expressions
By mastering algebraic manipulations, understanding special functions, working with mathematical constants, using calculus to simplify expressions, and practicing with real-world examples, you can unlock the secrets of closed form expressions. Remember to stay curious, persistent, and patient, and you will be well on your way to becoming a master of mathematical expressions.
Join the Conversation
We hope this article has provided you with a deeper understanding of closed form expressions and the five essential keys to unlocking their secrets. Share your thoughts and experiences with us in the comments below, and let's continue the conversation.
What is a closed form expression?
+A closed form expression is a mathematical expression that can be written in a finite and compact form, often using special functions or mathematical constants.
Why are closed form expressions important?
+Closed form expressions are essential in mathematics and science, as they provide a concise and elegant way to describe complex phenomena.
How can I simplify complex mathematical expressions?
+You can simplify complex mathematical expressions by mastering algebraic manipulations, understanding special functions, working with mathematical constants, using calculus to simplify expressions, and practicing with real-world examples.