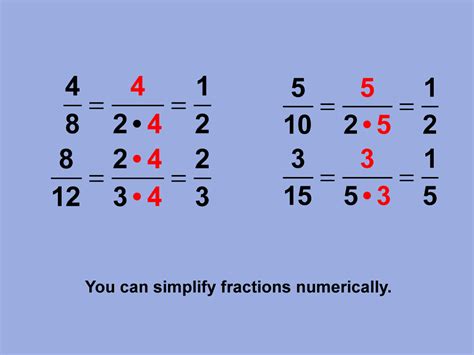
The concept of fractions is a fundamental aspect of mathematics, and simplifying them is a crucial skill to master. In this article, we will delve into the world of fractions, exploring what it means to simplify a fraction and how to do so. We will then apply this knowledge to the specific case of 20 as a fraction in simplest form.
Understanding Fractions

A fraction is a way to represent a part of a whole. It consists of two parts: the numerator, which represents the number of equal parts we have, and the denominator, which represents the total number of parts the whole is divided into. For example, in the fraction 3/4, the numerator is 3, and the denominator is 4.
What is Simplifying a Fraction?
Simplifying a fraction means reducing it to its lowest terms, where the numerator and denominator have no common factors other than 1. This is important because it makes the fraction easier to work with and understand. For instance, the fraction 6/8 can be simplified to 3/4, which is a more manageable and intuitive representation of the same value.
The Process of Simplifying a Fraction

To simplify a fraction, you need to find the greatest common divisor (GCD) of the numerator and the denominator. The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder. Once you have found the GCD, you divide both the numerator and the denominator by this number, resulting in a simplified fraction.
Example: Simplifying 20 as a Fraction
Let's say we want to express 20 as a fraction in simplest form. To do this, we first need to decide on a denominator. Since 20 is an integer, we can choose 1 as the denominator, making the fraction 20/1. However, this is not a very useful or simplified representation of 20 as a fraction.
A more meaningful approach would be to consider 20 as a fraction of a larger whole. For instance, if we think of 20 as part of a group of 100, we can express it as 20/100. However, this fraction is not in its simplest form because both the numerator and the denominator share a common factor of 20.
To simplify 20/100, we divide both the numerator and the denominator by 20, resulting in 1/5. This is a much simpler and more intuitive representation of the fraction.
Real-World Applications of Simplifying Fractions

Simplifying fractions has numerous real-world applications across various fields. In cooking, for example, recipes often involve fractions, and simplifying them can make it easier to scale up or down a recipe. In science, particularly in chemistry, fractional distillation is a process used to separate mixtures based on differences in boiling points, and understanding fractions is crucial for this process.
In finance, fractions are used to represent interest rates, investment returns, and other financial metrics. Simplifying these fractions can help investors and financial analysts make more informed decisions.
Conclusion and Call to Action
Simplifying fractions is an essential math skill with far-reaching applications. By understanding the process of simplifying fractions and applying it to real-world problems, you can enhance your problem-solving abilities and make more informed decisions.
We encourage you to practice simplifying fractions, starting with simple examples like 20 as a fraction. Share your experiences and any questions you may have in the comments below. Don't forget to like and share this article with your friends and family who might benefit from learning about fractions and their simplification.
FAQ Section:
What is the greatest common divisor (GCD)?
+The GCD is the largest number that divides both the numerator and the denominator of a fraction without leaving a remainder.
Why is simplifying fractions important?
+Simplifying fractions makes them easier to work with and understand, which is crucial in various real-world applications, including cooking, science, and finance.
How do I simplify a fraction?
+To simplify a fraction, find the GCD of the numerator and the denominator, then divide both numbers by the GCD.