The concept of 2 as a fraction in simplest form may seem straightforward, but it's an excellent opportunity to explore the fundamental principles of fractions and simplify complex numbers.
2 as a fraction can be written as 2/1. In this form, the number 2 is represented as a fraction with a numerator of 2 and a denominator of 1. This fraction is already in its simplest form, as the greatest common divisor (GCD) of 2 and 1 is 1.
However, understanding how to simplify fractions is essential in mathematics, particularly when dealing with more complex numbers. Let's dive into the world of fractions and explore how to simplify them.
What is a Fraction?

A fraction is a mathematical expression that represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number), separated by a horizontal line. Fractions can be used to represent a wide range of values, from simple proportions to complex ratios.
Types of Fractions
There are several types of fractions, including:
- Proper fractions: where the numerator is less than the denominator (e.g., 1/2, 3/4)
- Improper fractions: where the numerator is greater than or equal to the denominator (e.g., 2/1, 5/3)
- Mixed numbers: a combination of a whole number and a proper fraction (e.g., 2 1/2, 3 3/4)
Simplifying Fractions

Simplifying fractions involves reducing the numerator and denominator to their lowest terms, while maintaining the same value. To simplify a fraction, we need to find the greatest common divisor (GCD) of the numerator and denominator and divide both numbers by the GCD.
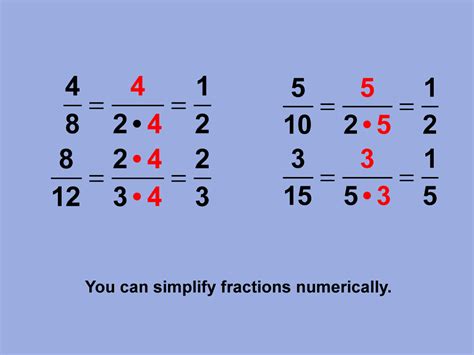
For example, let's simplify the fraction 4/8:
- Find the GCD of 4 and 8, which is 4.
- Divide both numbers by 4: 4 ÷ 4 = 1, 8 ÷ 4 = 2.
- The simplified fraction is 1/2.
Why Simplify Fractions?
Simplifying fractions is essential in mathematics because it helps to:
- Reduce confusion: by expressing fractions in their simplest form, we can avoid confusion and errors.
- Improve calculations: simplified fractions make calculations easier and more efficient.
- Enhance understanding: simplifying fractions helps to reveal the underlying relationships between numbers.
Real-World Applications of Fractions

Fractions have numerous real-world applications, including:
- Cooking: fractions are used to measure ingredients and scale recipes.
- Finance: fractions are used to calculate interest rates, investment returns, and financial ratios.
- Science: fractions are used to express proportions, concentrations, and ratios in scientific formulas.
In conclusion, understanding how to simplify fractions is a fundamental skill in mathematics. By grasping the concept of fractions and simplifying complex numbers, we can improve our calculations, enhance our understanding, and apply mathematical principles to real-world problems.
We invite you to share your thoughts on fractions and simplification in the comments below. Do you have a favorite method for simplifying fractions? Share your tips and tricks with our community!
What is the simplest form of the fraction 2/1?
+The simplest form of the fraction 2/1 is 2/1, as the GCD of 2 and 1 is 1.
How do you simplify a fraction?
+To simplify a fraction, find the GCD of the numerator and denominator and divide both numbers by the GCD.
What are some real-world applications of fractions?
+Fractions have numerous real-world applications, including cooking, finance, and science.