1.05 As A Fraction In Simplest Form

Quick Links :

Understanding Decimals and Fractions

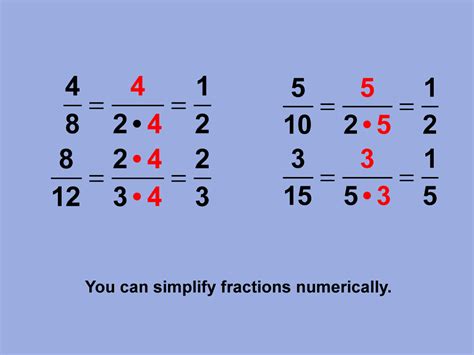
In the world of mathematics, decimals and fractions are two ways to represent the same value. While decimals are expressed as a number with a decimal point, fractions are represented as a ratio of two numbers. The conversion between decimals and fractions is a fundamental concept in mathematics, and it is essential to understand how to convert between these two forms.

For instance, the decimal 1.05 can be converted into a fraction. To do this, we need to find the ratio of the decimal value to the whole number value. In this case, 1.05 is equal to 105/100. However, this fraction can be simplified further.

Converting 1.05 to a Fraction

To convert 1.05 to a fraction, we can start by writing it as 105/100. This fraction can be simplified by dividing both the numerator and denominator by their greatest common divisor (GCD). The GCD of 105 and 100 is 5.

Simplifying the Fraction

By dividing both the numerator and denominator by 5, we get:

105 ÷ 5 = 21 100 ÷ 5 = 20

So, the simplified fraction of 1.05 is 21/20.

Verifying the Fraction

To verify that 21/20 is indeed the correct fraction for 1.05, we can convert it back to a decimal. To do this, we divide the numerator by the denominator:

21 ÷ 20 = 1.05

This confirms that 21/20 is the simplest form of the fraction for 1.05.

Benefits of Understanding Fractions

Understanding fractions is essential in various real-life applications, such as cooking, finance, and science. Fractions can help us to represent proportional relationships, make comparisons, and solve problems that involve proportional reasoning.

For instance, in cooking, recipes often require ingredients to be measured in fractions, such as 1/4 cup or 3/4 teaspoon. Understanding fractions can help us to adjust recipes, scale ingredients, and make conversions between different units.

In finance, fractions are used to represent interest rates, investment returns, and ratios of assets to liabilities. Understanding fractions can help us to make informed decisions about investments, loans, and credit.

In science, fractions are used to represent ratios of elements, mixtures, and compounds. Understanding fractions can help us to analyze data, make predictions, and draw conclusions about scientific phenomena.

Conclusion: Mastering Fractions and Decimals

In conclusion, understanding fractions and decimals is a fundamental concept in mathematics. By mastering the conversion between decimals and fractions, we can solve problems, make informed decisions, and communicate effectively in various real-life applications. The conversion of 1.05 to a fraction is a simple example of how to simplify decimals and represent them in their simplest form. By practicing and applying these concepts, we can become proficient in our ability to work with fractions and decimals.

We hope you found this article informative and helpful. If you have any questions or comments, please feel free to share them below.