Vertex Form Solver Made Easy

Quick Links :

The world of mathematics can be a daunting place, especially when dealing with complex equations and formulas. One such concept that can often leave students and professionals alike scratching their heads is the vertex form of a quadratic equation. But fear not, dear reader, for today we're going to break down the vertex form solver and make it easy to understand and work with.

In the realm of algebra, the vertex form of a quadratic equation is a powerful tool used to analyze and solve problems involving parabolas. Whether you're a student looking to ace your math exams or a professional seeking to apply mathematical concepts to real-world problems, mastering the vertex form solver is an essential skill to have. In this article, we'll delve into the world of vertex form solvers, exploring what they are, how they work, and providing practical examples to help solidify your understanding.

What is a Vertex Form Solver?

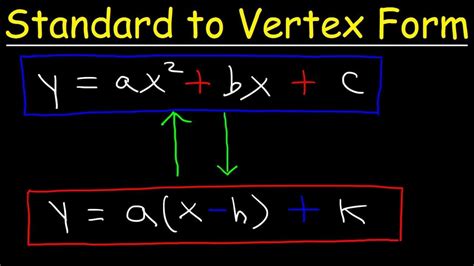
A vertex form solver is a mathematical tool used to solve quadratic equations in the form of y = a(x - h)^2 + k, where (h, k) represents the vertex of the parabola. The vertex form solver is a powerful tool that allows users to easily identify the vertex, axis of symmetry, and other key features of a parabola.

How Does a Vertex Form Solver Work?

A vertex form solver works by analyzing the coefficients of the quadratic equation and using them to calculate the vertex and other key features of the parabola. The process typically involves the following steps:

1. Identify the coefficients of the quadratic equation, including a, b, and c.
2. Use the coefficients to calculate the vertex (h, k) using the formulas h = -b / 2a and k = c - (b^2 / 4a).
3. Use the vertex to calculate the axis of symmetry, which is the vertical line that passes through the vertex.
4. Use the vertex and axis of symmetry to calculate other key features of the parabola, such as the x-intercepts and y-intercept.

Benefits of Using a Vertex Form Solver

Using a vertex form solver offers a number of benefits, including:

• Easy identification of the vertex and axis of symmetry
• Quick calculation of other key features of the parabola
• Simplified analysis of quadratic equations
• Improved accuracy and reduced errors

Practical Examples of Vertex Form Solvers

Let's take a look at a few practical examples of how to use a vertex form solver:

Example 1:

Find the vertex and axis of symmetry of the quadratic equation y = x^2 + 4x + 4.

Solution:

Using a vertex form solver, we can calculate the vertex as (h, k) = (-2, 0) and the axis of symmetry as x = -2.

Example 2:

Find the x-intercepts and y-intercept of the quadratic equation y = 2x^2 + 4x + 1.

Solution:

Using a vertex form solver, we can calculate the vertex as (h, k) = (-1, -1) and the axis of symmetry as x = -1. From this, we can calculate the x-intercepts as x = -1 ± √2 and the y-intercept as y = 1.

Common Applications of Vertex Form Solvers

Vertex form solvers have a wide range of applications in mathematics and real-world problems, including:

• Analyzing quadratic equations in algebra and calculus
• Modeling real-world phenomena, such as projectile motion and optimization problems
• Solving problems in physics, engineering, and economics

Conclusion

In conclusion, the vertex form solver is a powerful tool that can simplify the analysis of quadratic equations and make it easier to identify key features of parabolas. By understanding how to use a vertex form solver, you can improve your skills in algebra and calculus, and apply mathematical concepts to real-world problems.

Take Action

If you're looking to improve your math skills or apply mathematical concepts to real-world problems, we encourage you to try using a vertex form solver. With practice and patience, you can become proficient in using this powerful tool and take your math skills to the next level.