Vertex form is a fundamental concept in mathematics, particularly in algebra and geometry. It is a way of expressing a quadratic function in a specific format that provides valuable insights into the function's behavior and properties. In this article, we will delve into the definition and formula of vertex form in math, exploring its significance, applications, and examples.
What is Vertex Form?
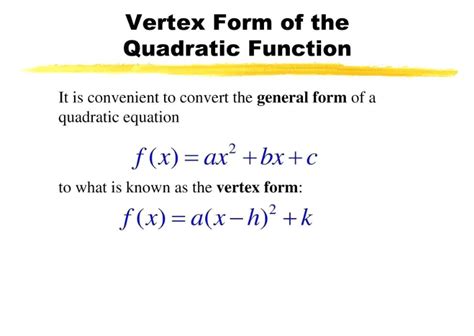
Vertex form is a way of representing a quadratic function in the form f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. The vertex is the lowest or highest point on the parabola, depending on the direction of the parabola's opening. The vertex form is also known as the standard form of a quadratic function.

Vertex Form Formula
The vertex form formula is given by:
f(x) = a(x - h)^2 + k
where:
- a is the coefficient of the squared term, which determines the direction and width of the parabola.
- h is the x-coordinate of the vertex, which represents the horizontal shift of the parabola.
- k is the y-coordinate of the vertex, which represents the vertical shift of the parabola.
Why is Vertex Form Important?
Vertex form is essential in mathematics and real-world applications because it provides a concise and intuitive way of expressing quadratic functions. By analyzing the vertex form, you can quickly identify the key features of the parabola, such as its vertex, axis of symmetry, and direction of opening. This information is crucial in various fields, including physics, engineering, economics, and computer science.
How to Convert Standard Form to Vertex Form
To convert a quadratic function from standard form to vertex form, you can follow these steps:
- Write the standard form of the quadratic function: f(x) = ax^2 + bx + c.
- Complete the square by adding and subtracting (b/2)^2 to the right-hand side of the equation.
- Factor the perfect square trinomial on the right-hand side of the equation.
- Write the resulting expression in vertex form: f(x) = a(x - h)^2 + k.

Examples of Vertex Form
Here are a few examples of quadratic functions in vertex form:
- f(x) = (x - 2)^2 + 3
- f(x) = -(x + 1)^2 - 2
- f(x) = 2(x - 3)^2 + 1
In each of these examples, the vertex form provides a clear and concise representation of the quadratic function, highlighting its key features and properties.
Applications of Vertex Form
Vertex form has numerous applications in various fields, including:
- Physics: Vertex form is used to model the trajectory of projectiles, such as the path of a thrown ball or the trajectory of a rocket.
- Engineering: Vertex form is used to design and optimize systems, such as bridges, buildings, and electronic circuits.
- Economics: Vertex form is used to model economic systems, such as supply and demand curves.
- Computer Science: Vertex form is used in computer graphics, game development, and machine learning.
Conclusion
In conclusion, vertex form is a powerful and versatile tool in mathematics, providing a concise and intuitive way of expressing quadratic functions. By understanding the definition, formula, and applications of vertex form, you can gain valuable insights into the behavior and properties of quadratic functions, which is essential in various fields and real-world applications.

We hope this article has provided you with a comprehensive understanding of vertex form in math. If you have any questions or comments, please feel free to share them below.
FAQ Section:
What is the vertex form of a quadratic function?
+The vertex form of a quadratic function is f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola.
How do I convert standard form to vertex form?
+To convert standard form to vertex form, complete the square by adding and subtracting (b/2)^2 to the right-hand side of the equation, and then factor the perfect square trinomial.
What are some applications of vertex form?
+Vertex form has numerous applications in physics, engineering, economics, and computer science, including modeling projectile motion, designing systems, and optimizing processes.