Complete The Square: Mastering Vertex Form Made Easy

Quick Links :

Hide

Completing the square is a fundamental concept in algebra that allows us to rewrite a quadratic equation in a specific form, known as vertex form. This form is incredibly useful for graphing parabolas, identifying key features, and solving equations. In this article, we will delve into the world of completing the square, exploring its benefits, mechanisms, and applications.

What is Completing the Square?

Completing the square is a mathematical technique used to rewrite a quadratic equation in the form (x - h)^2 + k = 0, where (h, k) represents the vertex of the parabola. This form is particularly useful for graphing parabolas, as it allows us to identify the vertex, axis of symmetry, and other key features.

Why is Completing the Square Important?

Completing the square is an essential tool in algebra, as it enables us to:

• Rewrite quadratic equations in a more convenient form
• Identify the vertex, axis of symmetry, and other key features of parabolas
• Solve quadratic equations and inequalities
• Graph parabolas and other quadratic functions
• Analyze and model real-world phenomena

The Mechanics of Completing the Square

Completing the square involves a series of steps that transform a quadratic equation into vertex form. Here's a step-by-step guide:

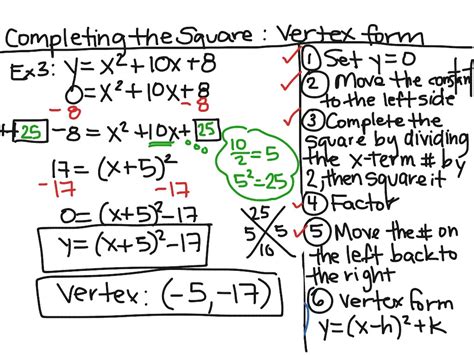
1. Start with a quadratic equation in the form ax^2 + bx + c = 0.
2. Move the constant term to the right-hand side: ax^2 + bx = -c.
3. Divide both sides by the coefficient of x^2 (a): x^2 + (b/a)x = -c/a.
4. Add and subtract (b/2a)^2 to the left-hand side: x^2 + (b/a)x + (b/2a)^2 - (b/2a)^2 = -c/a.
5. Factor the perfect square trinomial: (x + b/2a)^2 - (b/2a)^2 = -c/a.
6. Simplify the equation: (x + b/2a)^2 = -c/a + (b/2a)^2.

Example: Completing the Square

Let's complete the square for the quadratic equation x^2 + 6x + 8 = 0.

1. Move the constant term to the right-hand side: x^2 + 6x = -8.
2. Divide both sides by the coefficient of x^2 (1): x^2 + 6x = -8.
3. Add and subtract (6/2)^2 = 9 to the left-hand side: x^2 + 6x + 9 - 9 = -8.
4. Factor the perfect square trinomial: (x + 3)^2 - 9 = -8.
5. Simplify the equation: (x + 3)^2 = 1.

Applications of Completing the Square

Completing the square has numerous applications in mathematics, science, and engineering. Some of the most significant applications include:

• Graphing parabolas and other quadratic functions
• Solving quadratic equations and inequalities
• Analyzing and modeling real-world phenomena, such as projectile motion and optimization problems
• Calculating the area and volume of complex shapes

Real-World Example: Projectile Motion

A projectile is launched from the ground with an initial velocity of 20 m/s at an angle of 60° above the horizontal. Assuming negligible air resistance, calculate the maximum height reached by the projectile.

Using the equation for projectile motion, we can model the trajectory of the projectile as a quadratic function:

h(t) = -4.9t^2 + 20t + 0

Completing the square for this equation, we get:

h(t) = -(4.9)(t - 2.04)^2 + 20.4

From this equation, we can identify the vertex of the parabola as (2.04, 20.4), which represents the maximum height reached by the projectile.

Conclusion

Completing the square is a powerful tool in algebra that enables us to rewrite quadratic equations in a more convenient form, identify key features of parabolas, and solve equations and inequalities. By mastering this technique, we can analyze and model real-world phenomena, solve complex problems, and gain a deeper understanding of mathematical concepts.