The trigonometric form of complex numbers is a fundamental concept in mathematics, particularly in the fields of algebra, geometry, and calculus. It provides a unique way of representing complex numbers, making it easier to perform operations and understand their properties. In this article, we will delve into the world of trigonometric form of complex numbers, exploring its definition, benefits, and applications.
What is the Trigonometric Form of Complex Numbers?

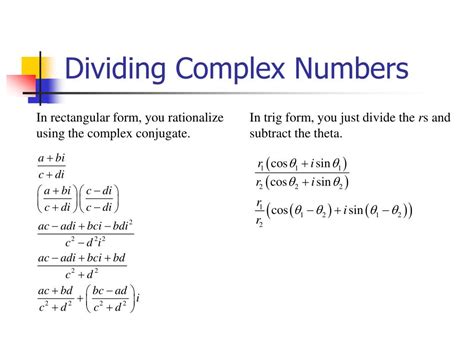
The trigonometric form of complex numbers is a way of expressing complex numbers using trigonometric functions, specifically sine and cosine. It is also known as the polar form of complex numbers. A complex number z can be represented in trigonometric form as:
z = r(cosθ + isinθ)
where r is the magnitude (or length) of the complex number, θ is the angle formed with the positive x-axis, and i is the imaginary unit.
Why is the Trigonometric Form Important?
The trigonometric form of complex numbers is essential in mathematics and science because it provides a powerful tool for solving problems involving complex numbers. Some of the benefits of using the trigonometric form include:
- Simplified calculations: The trigonometric form makes it easier to perform operations such as multiplication and division of complex numbers.
- Geometric interpretation: The trigonometric form provides a geometric interpretation of complex numbers, allowing us to visualize and understand their properties.
- Applications in science and engineering: The trigonometric form is used in many scientific and engineering applications, such as signal processing, control systems, and electromagnetism.
How to Convert Complex Numbers to Trigonometric Form

To convert a complex number to trigonometric form, we need to find the magnitude (r) and angle (θ) of the complex number. The following steps outline the conversion process:
- Find the magnitude (r) using the formula: r = √(x^2 + y^2)
- Find the angle (θ) using the formula: θ = arctan(y/x)
- Write the complex number in trigonometric form: z = r(cosθ + isinθ)
Examples of Converting Complex Numbers to Trigonometric Form
Here are a few examples of converting complex numbers to trigonometric form:
- Convert the complex number 3 + 4i to trigonometric form.
- Convert the complex number -2 - 5i to trigonometric form.
- Convert the complex number 1 + 1i to trigonometric form.
Applications of Trigonometric Form of Complex Numbers

The trigonometric form of complex numbers has numerous applications in science and engineering. Some of the key applications include:
- Signal processing: The trigonometric form is used to analyze and process signals in fields such as communication systems and audio processing.
- Control systems: The trigonometric form is used to design and analyze control systems, such as those used in robotics and aerospace engineering.
- Electromagnetism: The trigonometric form is used to describe the behavior of electromagnetic waves and to solve problems in electromagnetism.
Real-World Examples of Trigonometric Form of Complex Numbers
Here are a few real-world examples of the trigonometric form of complex numbers:
- In electrical engineering, the trigonometric form is used to analyze and design AC circuits.
- In signal processing, the trigonometric form is used to filter and process audio signals.
- In navigation, the trigonometric form is used to determine the position and velocity of objects.
Conclusion
In conclusion, the trigonometric form of complex numbers is a powerful tool for representing and working with complex numbers. Its importance lies in its ability to simplify calculations, provide geometric interpretation, and find applications in science and engineering. By understanding the trigonometric form of complex numbers, we can unlock the secrets of complex number arithmetic and apply them to real-world problems.
Now, we invite you to share your thoughts and experiences with complex numbers and trigonometric form. How have you used complex numbers in your studies or work? What are some of the challenges you have faced, and how have you overcome them? Share your comments and questions below.
What is the difference between the trigonometric form and the rectangular form of complex numbers?
+The trigonometric form and the rectangular form are two different ways of representing complex numbers. The rectangular form represents complex numbers as a + bi, where a and b are real numbers, while the trigonometric form represents complex numbers as r(cosθ + isinθ), where r is the magnitude and θ is the angle.
How do I convert a complex number from rectangular form to trigonometric form?
+To convert a complex number from rectangular form to trigonometric form, you need to find the magnitude (r) and angle (θ) of the complex number. The magnitude is found using the formula r = √(x^2 + y^2), and the angle is found using the formula θ = arctan(y/x).
What are some common applications of the trigonometric form of complex numbers?
+The trigonometric form of complex numbers has numerous applications in science and engineering, including signal processing, control systems, and electromagnetism.