Simplifying Square Root Of 96 In Radical Form

Quick Links :

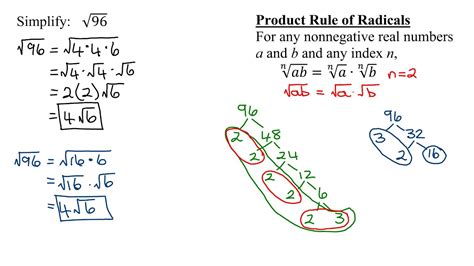
When working with radicals, it's essential to simplify them to their most basic form. The square root of 96 is a great example of a radical that can be simplified. In this article, we'll explore how to simplify the square root of 96 in radical form.

Understanding Radicals and Simplification

Radicals, also known as roots, are mathematical expressions that contain a square root, cube root, or other types of roots. Simplifying radicals involves expressing them in their most basic form, with no perfect squares or perfect cubes remaining under the radical sign.

The Importance of Simplifying Radicals

Simplifying radicals is crucial in mathematics, particularly in algebra and calculus. It helps to:

• Reduce complex expressions to their simplest form
• Make calculations easier and more efficient
• Enhance understanding of mathematical concepts
• Improve problem-solving skills

The Square Root of 96

The square root of 96 is expressed as √96. To simplify this radical, we need to find the largest perfect square that divides 96.

Prime Factorization of 96

To simplify the square root of 96, we'll use prime factorization. The prime factorization of 96 is:

96 = 2 × 2 × 2 × 2 × 2 × 3

Simplifying the Square Root of 96

Now, let's simplify the square root of 96 using the prime factorization:

√96 = √(2 × 2 × 2 × 2 × 2 × 3) = √(2^4 × 3) = 2^2 × √3 = 4√3

Therefore, the simplified form of the square root of 96 in radical form is 4√3.

Example Problems and Solutions

Here are some example problems and solutions to help you practice simplifying radicals:

• Example 1: Simplify √48 Solution: √48 = √(2 × 2 × 2 × 2 × 3) = 2^2 × √3 = 4√3
• Example 2: Simplify √75 Solution: √75 = √(3 × 5 × 5) = 5√3

Conclusion and Final Thoughts

Simplifying radicals is an essential skill in mathematics. By understanding the prime factorization of numbers and using simplification techniques, you can simplify complex radicals to their most basic form. The square root of 96 is a great example of a radical that can be simplified using prime factorization and simplification techniques.

We hope this article has helped you understand how to simplify the square root of 96 in radical form. Remember to practice simplifying radicals with different numbers to become proficient in this skill.

Final Tips and Recommendations

Here are some final tips and recommendations to help you master simplifying radicals:

• Practice simplifying radicals with different numbers and types of roots
• Use prime factorization to simplify radicals
• Check your work by plugging the simplified radical back into the original expression
• Use online resources and tutorials to supplement your learning

We encourage you to share your thoughts and questions in the comments section below. What are some common challenges you face when simplifying radicals? How do you think simplifying radicals can be applied in real-world problems?