Simplifying 7 28: The Easiest Way To Reduce Fractions

Quick Links :

Simplifying fractions is a fundamental concept in mathematics, and it's used in various aspects of life, from cooking to finance. Reducing fractions to their simplest form is essential to make calculations easier and more efficient. In this article, we will explore the easiest way to reduce fractions, and provide you with practical examples and tips to help you master this skill.

Understanding Fractions

Before we dive into simplifying fractions, let's review what fractions are. A fraction is a way to represent a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.

For example, the fraction 3/4 represents three equal parts out of a total of four parts.

Why Simplify Fractions?

Simplifying fractions is essential to make calculations easier and more efficient. When fractions are in their simplest form, it's easier to add, subtract, multiply, and divide them. Simplifying fractions also helps to avoid confusion and errors.

For instance, imagine you're baking a cake and the recipe calls for 3/4 cup of flour. If you have a 1/4 cup measuring cup, it's easier to measure 3/4 cup if the fraction is simplified.

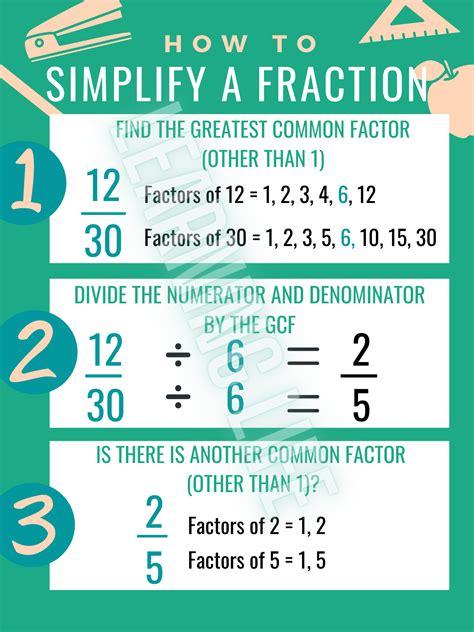
Step-by-Step Guide to Simplifying Fractions

Simplifying fractions is a straightforward process. Here's a step-by-step guide to help you simplify fractions:

1. Find the greatest common divisor (GCD): The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder.
2. Divide the numerator and denominator by the GCD: Once you've found the GCD, divide both the numerator and the denominator by this number.
3. Write the simplified fraction: The resulting fraction is the simplified form of the original fraction.

Let's use the fraction 6/8 as an example:

1. Find the GCD: The GCD of 6 and 8 is 2.
2. Divide the numerator and denominator by the GCD: 6 ÷ 2 = 3, and 8 ÷ 2 = 4.
3. Write the simplified fraction: The simplified fraction is 3/4.

Practical Examples and Tips

Here are some practical examples and tips to help you simplify fractions:

• Simplify fractions with variables: When simplifying fractions with variables, make sure to factor out any common factors.
• Use the canceling method: When multiplying fractions, you can cancel out common factors between the numerator and denominator.
• Check for equivalent ratios: When simplifying fractions, make sure to check for equivalent ratios.

For example, the fraction 2/4 can be simplified to 1/2, but the fraction 3/6 cannot be simplified further.

Common Mistakes to Avoid

Here are some common mistakes to avoid when simplifying fractions:

• Not finding the GCD: Make sure to find the GCD before simplifying fractions.
• Not dividing both numbers: Make sure to divide both the numerator and the denominator by the GCD.
• Not checking for equivalent ratios: Make sure to check for equivalent ratios when simplifying fractions.

By avoiding these common mistakes, you can ensure that you simplify fractions correctly.

Real-World Applications

Simplifying fractions has numerous real-world applications, from cooking to finance. Here are some examples:

• Cooking: Simplifying fractions is essential in cooking, especially when measuring ingredients.
• Finance: Simplifying fractions is used in finance to calculate interest rates and investments.
• Science: Simplifying fractions is used in science to calculate ratios and proportions.

By mastering the skill of simplifying fractions, you can make calculations easier and more efficient in various aspects of life.

Conclusion

Simplifying fractions is a fundamental concept in mathematics, and it's essential to make calculations easier and more efficient. By following the step-by-step guide and practical examples provided in this article, you can master the skill of simplifying fractions. Remember to avoid common mistakes and apply the skill in real-world applications.

We hope this article has helped you understand the easiest way to reduce fractions. Do you have any questions or comments about simplifying fractions? Share your thoughts in the comments section below.