The Sturm-Liouville form is a fundamental concept in mathematics, particularly in the field of differential equations. It is a mathematical framework that provides a powerful tool for solving various problems in physics, engineering, and other fields. In this article, we will delve into the world of Sturm-Liouville theory, exploring its definition, significance, and applications.
What is Sturm-Liouville Form?

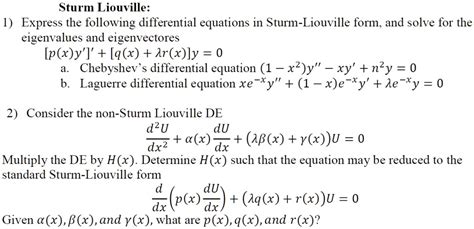
The Sturm-Liouville form is a specific type of differential equation that can be written in the following form:
p(x)y'' + q(x)y' + r(x)y = 0
where p(x), q(x), and r(x) are continuous functions on an interval [a, b]. The equation is named after the French mathematicians Jacques Charles François Sturm and Joseph Liouville, who first studied it in the mid-19th century.
Importance of Sturm-Liouville Form
The Sturm-Liouville form is essential in mathematics and physics because it provides a unified framework for solving various problems. It is a powerful tool for:
- Solving boundary value problems
- Analyzing the behavior of physical systems
- Studying the properties of differential operators
- Developing numerical methods for solving differential equations
Applications of Sturm-Liouville Form

The Sturm-Liouville form has numerous applications in various fields, including:
- Physics: The Schrödinger equation, which describes the behavior of quantum systems, is a Sturm-Liouville equation. The equation is also used to model the behavior of mechanical systems, such as vibrating strings and membranes.
- Engineering: The Sturm-Liouville form is used to analyze the behavior of electrical circuits, mechanical systems, and other engineering problems.
- Signal Processing: The Sturm-Liouville form is used in signal processing to analyze and filter signals.
- Computer Science: The Sturm-Liouville form is used in computer science to develop algorithms for solving differential equations and analyzing the behavior of complex systems.
Solving Sturm-Liouville Equations

Solving Sturm-Liouville equations involves finding the eigenvalues and eigenfunctions of the differential operator. The eigenvalues represent the frequencies of the system, while the eigenfunctions represent the modes of vibration.
The most common method for solving Sturm-Liouville equations is the separation of variables method. This method involves separating the equation into two ordinary differential equations, one for the spatial dependence and one for the temporal dependence.
Boundary Conditions
Boundary conditions play a crucial role in solving Sturm-Liouville equations. The boundary conditions determine the eigenvalues and eigenfunctions of the system. There are several types of boundary conditions, including:
- Dirichlet boundary conditions: The solution is specified at the boundary.
- Neumann boundary conditions: The derivative of the solution is specified at the boundary.
- Robin boundary conditions: A linear combination of the solution and its derivative is specified at the boundary.
Properties of Sturm-Liouville Equations

Sturm-Liouville equations have several important properties, including:
- Orthogonality: The eigenfunctions of the Sturm-Liouville equation are orthogonal to each other.
- Completeness: The eigenfunctions of the Sturm-Liouville equation form a complete set, meaning that any function can be expanded in terms of the eigenfunctions.
- Self-adjointness: The Sturm-Liouville operator is self-adjoint, meaning that it is equal to its own adjoint.
Conclusion and Future Directions
In conclusion, the Sturm-Liouville form is a powerful mathematical framework that provides a unified approach to solving various problems in physics, engineering, and other fields. The equation has numerous applications and is essential for understanding the behavior of complex systems.
Future research directions include developing new methods for solving Sturm-Liouville equations, such as numerical methods and asymptotic methods. Additionally, researchers are exploring new applications of the Sturm-Liouville form, such as in machine learning and data analysis.
We hope this article has provided a comprehensive overview of the Sturm-Liouville form and its applications. We encourage readers to share their thoughts and feedback in the comments section below.
What is the Sturm-Liouville form?
+The Sturm-Liouville form is a specific type of differential equation that can be written in the following form: p(x)y'' + q(x)y' + r(x)y = 0
What are the applications of the Sturm-Liouville form?
+The Sturm-Liouville form has numerous applications in physics, engineering, signal processing, and computer science.
How do you solve Sturm-Liouville equations?
+Solving Sturm-Liouville equations involves finding the eigenvalues and eigenfunctions of the differential operator. The most common method is the separation of variables method.