What Is 5 In Fraction Form Simplified

Quick Links :

The number 5 is a whole number, and as such, it can be represented as a fraction in its simplest form as 5/1.

In other words, the numerator (5) represents the whole number, and the denominator (1) represents the number of parts that make up the whole. Since 5 is already a whole number, the denominator is 1.

So, 5 in fraction form simplified is:

Now, let's dive deeper into the world of fractions and explore how we can work with them.

Understanding Fractions

A fraction is a way to represent a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.

For example, consider the fraction 3/4. Here, the numerator is 3, and the denominator is 4. This means we have 3 equal parts out of a total of 4 parts.

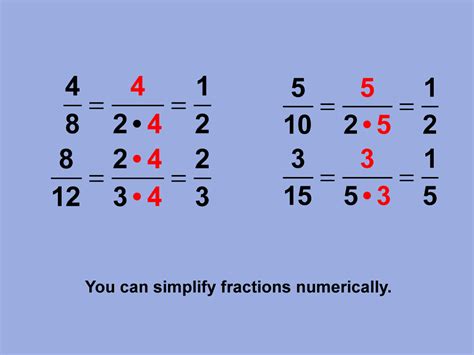
Simplifying Fractions

When working with fractions, it's often necessary to simplify them. Simplifying a fraction means reducing it to its lowest terms, where the numerator and denominator have no common factors other than 1.

To simplify a fraction, we need to find the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both numbers without leaving a remainder.

For example, consider the fraction 6/8. To simplify it, we need to find the GCD of 6 and 8, which is 2. We can then divide both the numerator and denominator by 2 to get:

6 ÷ 2 = 3 8 ÷ 2 = 4

So, the simplified fraction is 3/4.

Equivalent Fractions

Equivalent fractions are fractions that represent the same value. We can create equivalent fractions by multiplying or dividing both the numerator and denominator by the same number.

For example, consider the fraction 1/2. We can create an equivalent fraction by multiplying both the numerator and denominator by 2:

1 x 2 = 2 2 x 2 = 4

So, the equivalent fraction is 2/4.

Adding and Subtracting Fractions

When adding or subtracting fractions, we need to have the same denominator. If the denominators are different, we need to find the least common multiple (LCM) of the denominators.

The LCM is the smallest number that is a multiple of both denominators.

For example, consider the fractions 1/4 and 1/6. To add them, we need to find the LCM of 4 and 6, which is 12. We can then convert both fractions to have a denominator of 12:

1/4 = 3/12 1/6 = 2/12

Now, we can add the fractions:

3/12 + 2/12 = 5/12

Multiplying and Dividing Fractions

When multiplying fractions, we multiply the numerators and denominators separately:

(1/2) x (3/4) = 3/8

When dividing fractions, we invert the second fraction (i.e., flip the numerator and denominator) and then multiply:

(1/2) ÷ (3/4) = (1/2) x (4/3) = 4/6

Real-World Applications of Fractions

Fractions have many real-world applications in various fields, including:

• Cooking: Measuring ingredients for recipes
• Music: Representing rhythm and timing
• Finance: Calculating interest rates and investment returns
• Science: Measuring quantities and proportions

In conclusion, fractions are an essential part of mathematics, and understanding how to work with them is crucial for various real-world applications.

We hope this article has provided you with a comprehensive understanding of fractions and how to work with them.

Final Thoughts

In this article, we've explored the world of fractions and learned how to simplify, add, subtract, multiply, and divide them. We've also seen how fractions are used in real-world applications.

We encourage you to practice working with fractions and to explore their many uses in different fields.

If you have any questions or topics you'd like to discuss, please leave a comment below.