5 Ways To Convert Standard Form To Intercept Form Quadratic

Quick Links :

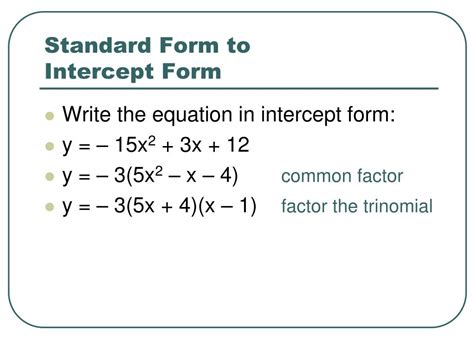
Converting standard form to intercept form quadratic is a fundamental skill in algebra, enabling you to analyze and solve quadratic equations more effectively. In this article, we will delve into the importance of converting standard form to intercept form, explore the benefits of doing so, and provide a step-by-step guide on how to accomplish this conversion using five distinct methods.

The standard form of a quadratic equation is given by ax^2 + bx + c = 0, where a, b, and c are constants. While this form is useful for many applications, it does not immediately reveal important information about the graph of the quadratic, such as the x-intercepts. Converting to intercept form, which is given by a(x - p)(x - q) = 0, allows us to easily identify the x-intercepts as p and q.

Why Convert Standard Form to Intercept Form?

Converting standard form to intercept form offers several advantages:

• Easy identification of x-intercepts: The intercept form allows you to directly read off the x-intercepts from the equation.
• Graphing: Knowing the x-intercepts makes it easier to graph the quadratic function.
• Solving quadratic equations: Intercept form can simplify the process of solving quadratic equations by factoring.

Now, let's explore the five methods for converting standard form to intercept form.

Method 1: Factoring

Factoring involves expressing the quadratic expression as a product of two binomials. This method is suitable when the quadratic expression can be easily factored.

Step-by-Step Process:

1. Start with the standard form of the quadratic equation: ax^2 + bx + c = 0.
2. Look for two numbers whose product is ac and whose sum is b. These numbers will be used to rewrite the middle term.
3. Rewrite the quadratic expression as a product of two binomials.
4. Set each binomial equal to zero and solve for x to find the x-intercepts.

Example:

Convert x^2 + 5x + 6 = 0 to intercept form using factoring.

Solution:

1. Rewrite the middle term: x^2 + 5x + 6 = (x + 2)(x + 3) = 0.
2. Set each binomial equal to zero: x + 2 = 0 or x + 3 = 0.
3. Solve for x: x = -2 or x = -3.

The intercept form is (x + 2)(x + 3) = 0.

Method 2: Quadratic Formula

The quadratic formula is a general method for solving quadratic equations. It can be used to convert standard form to intercept form.

Step-by-Step Process:

1. Use the quadratic formula: x = (-b ± √(b^2 - 4ac)) / 2a.
2. Simplify the expression under the square root.
3. Rewrite the quadratic expression in intercept form using the solutions.

Example:

Convert x^2 + 4x + 4 = 0 to intercept form using the quadratic formula.

Solution:

1. Apply the quadratic formula: x = (-4 ± √(4^2 - 4(1)(4))) / 2(1).
2. Simplify the expression: x = (-4 ± √(16 - 16)) / 2.
3. Rewrite in intercept form: (x + 2)(x + 2) = 0.

Method 3: Completing the Square

Completing the square is another method for converting standard form to intercept form.

Step-by-Step Process:

1. Move the constant term to the right side: x^2 + bx = -c.
2. Add (b/2)^2 to both sides to complete the square.
3. Rewrite the left side as a perfect square trinomial.
4. Set the perfect square trinomial equal to zero and solve for x.

Example:

Convert x^2 + 6x + 9 = 0 to intercept form using completing the square.

Solution:

1. Move the constant term: x^2 + 6x = -9.
2. Complete the square: x^2 + 6x + 9 = -9 + 9.
3. Rewrite the left side: (x + 3)^2 = 0.
4. Solve for x: x = -3.

The intercept form is (x + 3)^2 = 0.

Method 4: Graphing

Graphing is a visual method for converting standard form to intercept form.

Step-by-Step Process:

1. Graph the quadratic function.
2. Identify the x-intercepts from the graph.
3. Write the intercept form using the x-intercepts.

Example:

Convert x^2 - 4x - 3 = 0 to intercept form using graphing.

Solution:

1. Graph the quadratic function.
2. Identify the x-intercepts: x = -1 or x = 3.
3. Write the intercept form: (x + 1)(x - 3) = 0.

Method 5: Synthetic Division

Synthetic division is a method for dividing polynomials. It can be used to convert standard form to intercept form.

Step-by-Step Process:

1. Divide the quadratic expression by a linear factor.
2. Identify the quotient and remainder.
3. Write the intercept form using the quotient and remainder.

Example:

Convert x^2 + 2x - 6 = 0 to intercept form using synthetic division.

Solution:

1. Divide the quadratic expression by x + 3.
2. Identify the quotient and remainder: x - 2 with a remainder of 0.
3. Write the intercept form: (x + 3)(x - 2) = 0.

Now that we have explored the five methods for converting standard form to intercept form, let's summarize the key points.

• Factoring: Suitable when the quadratic expression can be easily factored.
• Quadratic Formula: A general method for solving quadratic equations.
• Completing the Square: Involves adding (b/2)^2 to both sides to complete the square.
• Graphing: A visual method that involves graphing the quadratic function.
• Synthetic Division: A method for dividing polynomials.

By mastering these five methods, you can effectively convert standard form to intercept form, making it easier to analyze and solve quadratic equations.

What method do you think is the most effective for converting standard form to intercept form? Share your thoughts and questions in the comments below!

Share this article with your friends and colleagues who may find it useful.