Understanding The Standard Form Of An Ellipse Equation

Quick Links :

The standard form of an ellipse equation is a fundamental concept in mathematics, particularly in geometry and algebra. An ellipse is a type of closed curve that is symmetrical about two axes, known as the major and minor axes. Understanding the standard form of an ellipse equation is essential for solving problems involving ellipses, such as finding the area, perimeter, and equation of an ellipse.

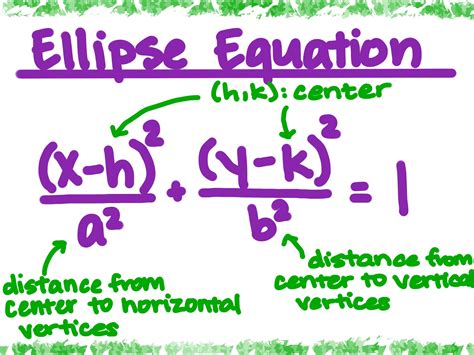
The standard form of an ellipse equation is given by:

(x - h)^2/a^2 + (y - k)^2/b^2 = 1

where (h, k) is the center of the ellipse, a is the semi-major axis, and b is the semi-minor axis.

Breaking Down The Standard Form Of An Ellipse Equation

To understand the standard form of an ellipse equation, it is essential to break down the equation into its components.

The Center Of The Ellipse

The center of the ellipse is given by (h, k). This point is the midpoint of the major and minor axes.

The Semi-Major Axis

The semi-major axis is given by a. This value represents the distance from the center of the ellipse to the farthest point on the ellipse along the major axis.

The Semi-Minor Axis

The semi-minor axis is given by b. This value represents the distance from the center of the ellipse to the farthest point on the ellipse along the minor axis.

Deriving The Standard Form Of An Ellipse Equation

The standard form of an ellipse equation can be derived using the definition of an ellipse. An ellipse is defined as the set of all points (x, y) in a plane such that the sum of the distances from two fixed points (foci) is constant.

Using the distance formula, the equation of an ellipse can be written as:

√((x - f1)^2 + (y - f2)^2) + √((x - f2)^2 + (y - f1)^2) = 2a

where (f1, f2) are the foci of the ellipse.

By expanding and simplifying the equation, we can derive the standard form of an ellipse equation:

(x - h)^2/a^2 + (y - k)^2/b^2 = 1

Graphing An Ellipse Using The Standard Form Equation

Graphing an ellipse using the standard form equation is a straightforward process. The center of the ellipse is given by (h, k), and the semi-major and semi-minor axes are given by a and b, respectively.

To graph an ellipse, we can use the following steps:

1. Plot the center of the ellipse at (h, k).
2. Plot the semi-major axis along the x-axis, starting from the center of the ellipse.
3. Plot the semi-minor axis along the y-axis, starting from the center of the ellipse.
4. Use the standard form equation to find the coordinates of the ellipse.

Applications Of The Standard Form Of An Ellipse Equation

The standard form of an ellipse equation has numerous applications in mathematics, physics, engineering, and other fields. Some of the applications include:

1. Architecture: Ellipses are used in the design of buildings, bridges, and other structures.
2. Physics: Ellipses are used to model the orbits of planets, comets, and other celestial bodies.
3. Engineering: Ellipses are used in the design of roads, tunnels, and other infrastructure projects.

Example 1: Finding The Equation Of An Ellipse

Find the equation of an ellipse with center (2, 3), semi-major axis 5, and semi-minor axis 3.

Using the standard form equation, we can write:

(x - 2)^2/5^2 + (y - 3)^2/3^2 = 1

Example 2: Graphing An Ellipse

Graph the ellipse with equation:

(x - 1)^2/4^2 + (y - 2)^2/2^2 = 1

Using the standard form equation, we can graph the ellipse as follows:

1. Plot the center of the ellipse at (1, 2).
2. Plot the semi-major axis along the x-axis, starting from the center of the ellipse.
3. Plot the semi-minor axis along the y-axis, starting from the center of the ellipse.

We hope this article has helped you understand the standard form of an ellipse equation. Do you have any questions or comments? Share your thoughts below!