Ellipse calculations can be a daunting task, especially when dealing with complex equations and formulas. However, with the right tools and resources, calculating ellipses can become a breeze. One such tool is the standard form ellipse calculator, which simplifies the process of calculating ellipse properties.
An ellipse is a curve on a plane surrounding two focal points such that the sum of the distances to the two focal points is constant. Ellipses have numerous applications in various fields, including physics, engineering, and mathematics. They are used to model real-world phenomena, such as the orbits of planets, the shape of mirrors, and the design of architectural structures.
In this article, we will explore the standard form ellipse calculator, its benefits, and how to use it for easy curve calculations.
What is a Standard Form Ellipse Calculator?

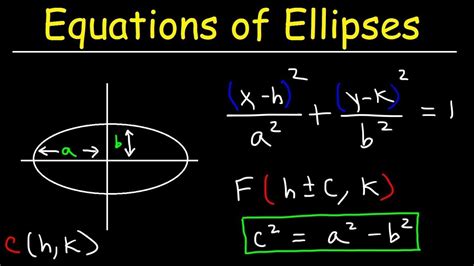
A standard form ellipse calculator is an online tool that calculates the properties of an ellipse, given its major and minor axes, or its semi-major and semi-minor axes. The calculator uses the standard form of an ellipse equation, which is:
(x^2/a^2) + (y^2/b^2) = 1
where (x, y) is a point on the ellipse, a is the semi-major axis, and b is the semi-minor axis.
Benefits of Using a Standard Form Ellipse Calculator
Using a standard form ellipse calculator offers several benefits, including:
- Saves time: Calculating ellipse properties manually can be time-consuming and prone to errors. A standard form ellipse calculator saves time and effort by providing instant results.
- Improves accuracy: The calculator ensures accurate calculations, reducing the likelihood of human error.
- Easy to use: Standard form ellipse calculators are designed to be user-friendly, making it easy to input values and obtain results.
How to Use a Standard Form Ellipse Calculator

Using a standard form ellipse calculator is straightforward. Here's a step-by-step guide:
- Enter the values: Input the values of the semi-major axis (a) and semi-minor axis (b) into the calculator.
- Choose the calculation: Select the property you want to calculate, such as the area, perimeter, or focal distance.
- Get the result: The calculator will display the result instantly.
Properties of an Ellipse
An ellipse has several properties that can be calculated using a standard form ellipse calculator. Some of these properties include:
- Area: The area of an ellipse is given by the formula: A = πab
- Perimeter: The perimeter of an ellipse is approximately equal to: P ≈ π[3(a+b) - √((3a+b)(a+3b))]
- Focal distance: The focal distance is the distance between the two focal points and is given by: c = √(a^2 - b^2)
Applications of Ellipse Calculations

Ellipse calculations have numerous applications in various fields, including:
- Physics: Ellipses are used to model the orbits of planets, the shape of mirrors, and the design of optical systems.
- Engineering: Ellipses are used in the design of architectural structures, such as bridges, tunnels, and buildings.
- Mathematics: Ellipses are used to study geometry, trigonometry, and calculus.
Real-World Examples of Ellipse Calculations
- Designing a satellite dish: Ellipse calculations are used to design the shape of a satellite dish, which is typically an ellipse.
- Modeling the orbit of a planet: Ellipse calculations are used to model the orbit of a planet, such as the Earth's orbit around the Sun.
- Designing a stadium: Ellipse calculations are used to design the shape of a stadium, such as a football stadium.
Conclusion
A standard form ellipse calculator is a powerful tool that simplifies the process of calculating ellipse properties. With its ease of use and accuracy, it's an essential tool for anyone working with ellipses. Whether you're a student, engineer, or mathematician, a standard form ellipse calculator can help you with your ellipse calculations.

We hope this article has provided you with a comprehensive understanding of standard form ellipse calculators and their applications. If you have any questions or comments, please feel free to share them below.
What is the standard form of an ellipse equation?
+The standard form of an ellipse equation is (x^2/a^2) + (y^2/b^2) = 1, where (x, y) is a point on the ellipse, a is the semi-major axis, and b is the semi-minor axis.
What are the benefits of using a standard form ellipse calculator?
+The benefits of using a standard form ellipse calculator include saving time, improving accuracy, and ease of use.
What are some real-world examples of ellipse calculations?
+Real-world examples of ellipse calculations include designing a satellite dish, modeling the orbit of a planet, and designing a stadium.