Standard and expanded form are two essential concepts in mathematics that help students understand the relationship between numbers and their place value. Mastering these concepts is crucial for building a strong foundation in math, as they are used extensively in various mathematical operations, such as addition, subtraction, multiplication, and division.
In this article, we will delve into the world of standard and expanded form, exploring their definitions, benefits, and practical applications. We will also provide examples, tips, and resources to help you master these concepts and become a math whiz.
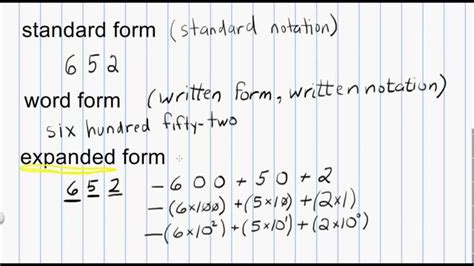
What is Standard Form in Math?

Standard form, also known as the standard notation, is a way of expressing numbers in a concise and readable format. It is the most common way of writing numbers in math, and it involves expressing a number in terms of its digits and their corresponding place values. In standard form, each digit is multiplied by a power of 10, and the results are added together to form the final number.
For example, the number 456 can be expressed in standard form as:
4 × 10^2 + 5 × 10^1 + 6 × 10^0
This notation helps to clarify the relationship between each digit and its place value, making it easier to perform mathematical operations.
Benefits of Standard Form
Standard form has several benefits that make it an essential concept in math:
- Improved readability: Standard form makes numbers easier to read and understand, especially when dealing with large numbers.
- Simplified calculations: Standard form simplifies mathematical operations, such as addition and subtraction, by allowing you to focus on each digit and its corresponding place value.
- Enhanced accuracy: Standard form reduces errors by making it clear which digit corresponds to which place value.
What is Expanded Form in Math?

Expanded form is another way of expressing numbers in math, and it involves breaking down a number into its individual digits and their corresponding place values. Unlike standard form, expanded form shows the actual value of each digit, making it easier to understand the concept of place value.
For example, the number 456 can be expressed in expanded form as:
400 + 50 + 6
This notation helps to illustrate the concept of place value, making it easier to understand how numbers are constructed.
Benefits of Expanded Form
Expanded form has several benefits that make it an essential concept in math:
- Improved understanding of place value: Expanded form helps to illustrate the concept of place value, making it easier to understand how numbers are constructed.
- Enhanced visualization: Expanded form provides a visual representation of numbers, making it easier to understand the relationship between each digit and its corresponding place value.
- Simplified calculations: Expanded form simplifies mathematical operations, such as addition and subtraction, by allowing you to focus on each digit and its corresponding place value.
Practical Applications of Standard and Expanded Form
Standard and expanded form have numerous practical applications in math, including:
- Addition and subtraction: Standard and expanded form are used extensively in addition and subtraction operations, making it easier to perform calculations.
- Multiplication and division: Standard and expanded form are used in multiplication and division operations, making it easier to perform calculations.
- Real-world applications: Standard and expanded form are used in real-world applications, such as finance, science, and engineering, to perform calculations and solve problems.
Tips for Mastering Standard and Expanded Form
Here are some tips for mastering standard and expanded form:
- Practice, practice, practice: Practice is key to mastering standard and expanded form. Practice converting numbers between standard and expanded form to become more comfortable with these concepts.
- Use visual aids: Visual aids, such as diagrams and charts, can help to illustrate the concept of place value and make it easier to understand standard and expanded form.
- Focus on place value: Focus on the concept of place value, as it is essential to understanding standard and expanded form.
Conclusion
Mastering standard and expanded form is essential for building a strong foundation in math. By understanding the concepts of standard and expanded form, you can improve your math skills and become more confident in your ability to perform mathematical operations.
What is the difference between standard form and expanded form?
+Standard form is a way of expressing numbers in a concise and readable format, while expanded form is a way of breaking down a number into its individual digits and their corresponding place values.
How do I convert a number from standard form to expanded form?
+To convert a number from standard form to expanded form, you need to break down the number into its individual digits and their corresponding place values. For example, the number 456 can be converted to expanded form as 400 + 50 + 6.
What are the benefits of using standard and expanded form?
+The benefits of using standard and expanded form include improved readability, simplified calculations, and enhanced accuracy. Standard and expanded form also help to illustrate the concept of place value, making it easier to understand how numbers are constructed.
We hope this article has helped you to understand the concepts of standard and expanded form and how to apply them in math. Do you have any questions or comments about standard and expanded form? Share your thoughts in the comments section below!