The concept of square roots is fundamental in mathematics, particularly in algebra and geometry. The square root of a number is a value that, when multiplied by itself, gives the original number. In this article, we will explore the square root of 98 in radical form simplified.
What is the Square Root of 98?
The square root of 98 can be calculated using a calculator or estimated using mental math tricks. However, in radical form, we can simplify it by finding perfect square factors.
Finding Perfect Square Factors
To simplify the square root of 98, we need to find perfect square factors. A perfect square is a number that can be expressed as the product of an integer with itself. For example, 16 is a perfect square because it can be expressed as 4 × 4.
The prime factorization of 98 is 2 × 7 × 7. We can see that 7 is repeated twice, which means it is a perfect square.
Simplifying the Square Root of 98
Using the perfect square factor, we can simplify the square root of 98 as follows:
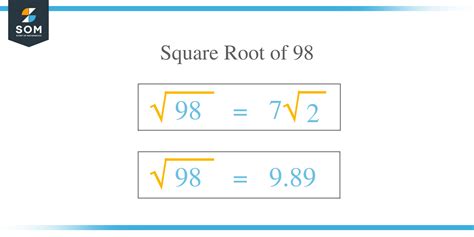
√98 = √(2 × 7 × 7) = √(2) × √(7 × 7) = √2 × 7
So, the square root of 98 in radical form simplified is √2 × 7.

How to Simplify Radical Expressions
Simplifying radical expressions involves finding perfect square factors and combining them with the square root symbol. Here are the general steps:
- Find the prime factorization of the number inside the square root.
- Identify perfect square factors by looking for repeated prime factors.
- Combine the perfect square factors with the square root symbol.
- Simplify the expression by multiplying the perfect square factors.
Example: Simplifying the Square Root of 48
The prime factorization of 48 is 2 × 2 × 2 × 2 × 3. We can see that 2 is repeated four times, which means it is a perfect square.
√48 = √(2 × 2 × 2 × 2 × 3) = √(2 × 2) × √(2 × 2) × √3 = 4 × √3
So, the square root of 48 in radical form simplified is 4√3.
Benefits of Simplifying Radical Expressions
Simplifying radical expressions has several benefits:
- It makes calculations easier and faster.
- It helps to identify patterns and relationships between numbers.
- It provides a clearer understanding of mathematical concepts and principles.
Real-World Applications of Radical Expressions
Radical expressions have numerous real-world applications in physics, engineering, and computer science. Here are a few examples:
- Calculating distances and velocities in physics
- Designing electronic circuits and filters
- Modeling population growth and epidemiology
Conclusion
In conclusion, the square root of 98 in radical form simplified is √2 × 7. Simplifying radical expressions involves finding perfect square factors and combining them with the square root symbol. This process has numerous benefits, including easier calculations, pattern recognition, and a deeper understanding of mathematical concepts. Radical expressions also have real-world applications in various fields, making them an essential tool for problem-solving and critical thinking.
What's Next?
If you're interested in learning more about radical expressions and their applications, we recommend exploring online resources and textbooks on algebra and mathematics. You can also practice simplifying radical expressions using online calculators or worksheets.
Share Your Thoughts!
Do you have any questions or comments about simplifying radical expressions? Share your thoughts and experiences in the comments section below. We'd love to hear from you!
FAQ Section:
What is the square root of 98 in decimal form?
+The square root of 98 in decimal form is approximately 9.8995.
How do I simplify radical expressions with variables?
+To simplify radical expressions with variables, follow the same steps as simplifying radical expressions with numbers. Look for perfect square factors and combine them with the square root symbol.
What are some real-world applications of radical expressions in physics?
+Radical expressions are used in physics to calculate distances, velocities, and accelerations. They are also used to model population growth and epidemiology.