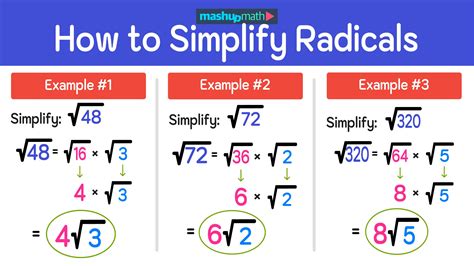
Simplifying radicals can be a daunting task, but with the right approach, it can be done in just one step. In this article, we will explore how to simplify the square root of 45 in a single step.
Understanding Radicals and Their Simplification

Radicals are mathematical expressions that contain a root symbol (√) and a number or expression inside the radical sign. The most common type of radical is the square root (√), which is used to find the number that, when multiplied by itself, gives the original value. Simplifying radicals involves expressing the radical in its simplest form, which can be achieved by factoring the number inside the radical sign.
Why Simplify Radicals?
Simplifying radicals is essential in mathematics because it helps to:
- Make calculations easier and more efficient
- Reduce the complexity of mathematical expressions
- Improve understanding and visualization of mathematical concepts
Simplifying the Square Root of 45 in 1 Step

Now, let's simplify the square root of 45 in just one step. To do this, we need to find the largest perfect square that is a factor of 45.
√45 = √(9 × 5)
Since 9 is a perfect square (3 × 3), we can simplify the radical as follows:
√45 = √(9 × 5) = √9 × √5 = 3√5
And that's it! We have simplified the square root of 45 in just one step.
Key Takeaway
When simplifying radicals, it's essential to look for perfect squares that are factors of the number inside the radical sign. By factoring out the perfect square, we can simplify the radical and make calculations easier.
Benefits of Simplifying Radicals

Simplifying radicals has numerous benefits in mathematics, including:
- Simplifying complex expressions and equations
- Making calculations faster and more efficient
- Improving understanding and visualization of mathematical concepts
- Enhancing problem-solving skills
Practical Applications of Radical Simplification
Radical simplification has many practical applications in mathematics, science, and engineering, including:
- Calculating distances and velocities in physics
- Solving quadratic equations in algebra
- Modeling population growth and decay in biology
- Optimizing functions in calculus
Conclusion and Next Steps

In this article, we have explored how to simplify the square root of 45 in just one step. By factoring out the perfect square, we can simplify the radical and make calculations easier. Remember, simplifying radicals is an essential skill in mathematics, and it has many practical applications in science, engineering, and other fields.
What's your experience with simplifying radicals? Share your thoughts and questions in the comments below!
What is the purpose of simplifying radicals?
+Simplifying radicals helps to make calculations easier and more efficient, reduces the complexity of mathematical expressions, and improves understanding and visualization of mathematical concepts.
How do I simplify the square root of a number?
+To simplify the square root of a number, look for perfect squares that are factors of the number inside the radical sign. By factoring out the perfect square, you can simplify the radical and make calculations easier.
What are some practical applications of radical simplification?
+Retail simplification has many practical applications in mathematics, science, and engineering, including calculating distances and velocities in physics, solving quadratic equations in algebra, modeling population growth and decay in biology, and optimizing functions in calculus.