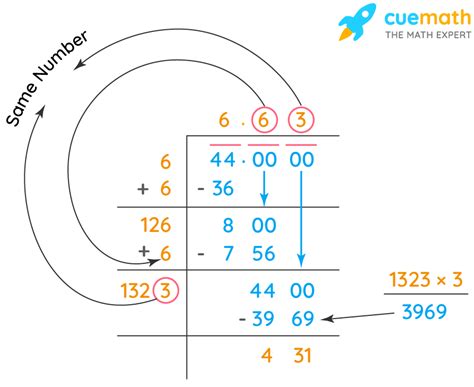
Simplifying the square root of 44 is a fundamental concept in mathematics, particularly in algebra and geometry. The square root of a number is a value that, when multiplied by itself, gives the original number. In this case, we are looking to simplify the square root of 44, which can be denoted as √44.
For many students, simplifying square roots can be a daunting task, especially when dealing with larger numbers like 44. However, with a few simple steps and an understanding of the underlying mathematical principles, anyone can learn to simplify the square root of 44.

What is the Square Root of 44?
Before we dive into simplifying the square root of 44, let's first understand what the square root of 44 actually is. The square root of 44 is a value that, when multiplied by itself, gives the result of 44. In mathematical terms, this can be expressed as:
√44 = x
where x is the value we are trying to find.
Using Prime Factorization to Simplify the Square Root of 44
One of the most effective ways to simplify the square root of 44 is to use prime factorization. Prime factorization involves breaking down a number into its prime factors, which are the prime numbers that multiply together to give the original number.
In the case of 44, the prime factorization is:
44 = 2 × 2 × 11
Now, let's take the square root of both sides of the equation:
√44 = √(2 × 2 × 11)
Using the properties of square roots, we can simplify this expression:
√44 = √(2^2) × √11
Since the square root of 2^2 is simply 2, we can further simplify the expression:
√44 = 2√11

Why is Simplifying the Square Root of 44 Important?
Simplifying the square root of 44 is important for a variety of reasons. Firstly, it helps to build a strong foundation in mathematics, particularly in algebra and geometry. By understanding how to simplify square roots, students can develop a deeper understanding of mathematical concepts and build a strong foundation for future learning.
Secondly, simplifying the square root of 44 is a crucial skill in many real-world applications, such as engineering, physics, and computer science. In these fields, square roots are used to calculate distances, velocities, and frequencies, making it essential to be able to simplify them accurately.
Real-World Applications of Simplifying the Square Root of 44
Simplifying the square root of 44 has many real-world applications, including:
- Calculating distances and velocities in physics and engineering
- Determining frequencies and wavelengths in physics and computer science
- Solving equations and inequalities in algebra and geometry
- Analyzing data and making predictions in statistics and data analysis

Conclusion and Final Thoughts
In conclusion, simplifying the square root of 44 is an essential mathematical concept that has many real-world applications. By using prime factorization and understanding the underlying mathematical principles, anyone can learn to simplify the square root of 44.
Whether you are a student looking to build a strong foundation in mathematics or a professional looking to apply mathematical concepts to real-world problems, simplifying the square root of 44 is a skill that is well worth learning.

What is the square root of 44?
+The square root of 44 is √44 = 2√11.
Why is simplifying the square root of 44 important?
+Simplifying the square root of 44 is important because it helps build a strong foundation in mathematics and has many real-world applications in fields such as engineering, physics, and computer science.
What are some real-world applications of simplifying the square root of 44?
+Some real-world applications of simplifying the square root of 44 include calculating distances and velocities in physics and engineering, determining frequencies and wavelengths in physics and computer science, and solving equations and inequalities in algebra and geometry.