3 Easy Ways To Express √36 In Radical Form

Quick Links :

Hide

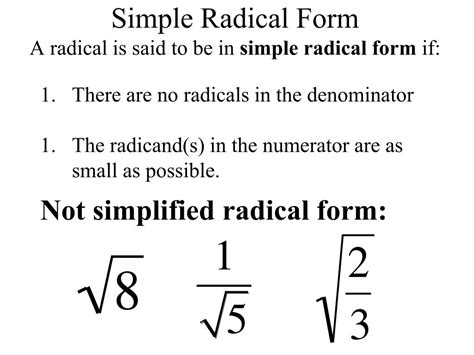
Expressing square roots in radical form can be a bit tricky, but don't worry, we've got you covered. In this article, we'll explore three easy ways to express √36 in radical form.

First, let's understand what radical form means. Radical form refers to the way we write square roots using the radical symbol (√). It's a way to represent the square root of a number in a more simplified and elegant form. Now, let's dive into the three easy ways to express √36 in radical form.

Method 1: Finding the Perfect Square

One way to express √36 in radical form is to find the perfect square that divides 36. A perfect square is a number that can be expressed as the square of an integer. In this case, 36 can be expressed as 6^2. Since 6^2 = 36, we can write √36 as √(6^2).

Using the property of square roots, we can simplify this expression to √(6^2) = 6. Therefore, one way to express √36 in radical form is simply as 6.

Example:

√36 = √(6^2) = 6

Method 2: Factoring the Radicand

Another way to express √36 in radical form is to factor the radicand (the number inside the square root). In this case, we can factor 36 as 36 = 6 × 6.

Using the property of square roots, we can write √36 as √(6 × 6). Since the square root of a product is equal to the product of the square roots, we can simplify this expression to √(6 × 6) = √6 × √6.

Therefore, another way to express √36 in radical form is as √6 × √6 or 6√6.

Example:

√36 = √(6 × 6) = √6 × √6 = 6√6

Method 3: Using the Square Root Property

The third way to express √36 in radical form is to use the square root property. This property states that √(a^2) = a, where a is a non-negative real number.

In this case, we can express 36 as 6^2. Using the square root property, we can write √36 as √(6^2). Since √(a^2) = a, we can simplify this expression to √(6^2) = 6.

Therefore, a third way to express √36 in radical form is simply as 6.

Example:

√36 = √(6^2) = 6

Comparison of Methods

All three methods yield the same result: √36 can be expressed as 6 or 6√6 in radical form. However, method 1 is the simplest and most straightforward approach, while method 2 provides a more detailed explanation of the factoring process. Method 3 uses the square root property to simplify the expression.

Conclusion

Expressing √36 in radical form is a simple process that can be achieved using three different methods. Whether you find the perfect square, factor the radicand, or use the square root property, the result is the same: √36 can be expressed as 6 or 6√6 in radical form. By understanding these methods, you can simplify square roots and express them in a more elegant and radical form.

Practice Problems:

1. Express √25 in radical form using method 1.
2. Express √49 in radical form using method 2.
3. Express √64 in radical form using method 3.

We hope this article has helped you understand how to express √36 in radical form. Do you have any questions or need further clarification? Leave a comment below or share this article with your friends!