Square Root Of 29 In Radical Form Simplified

Quick Links :

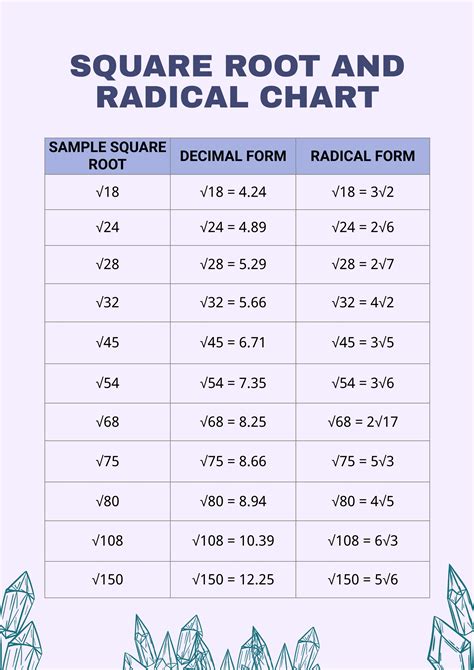
The concept of square roots is a fundamental aspect of mathematics, particularly in algebra and geometry. It represents the value that, when multiplied by itself, gives the original number. For instance, the square root of 16 is 4 because 4 multiplied by 4 equals 16. However, not all numbers have a whole number as their square root. Some numbers, like 29, do not have a perfect square root. Instead, their square roots are expressed in radical form.

Radical form is a mathematical notation that represents the square root of a number using the radical symbol (√). It signifies that the number inside the radical is the radicand, and the result is the square root of that radicand. The square root of 29 is an example of an irrational number because it cannot be expressed as a finite decimal or fraction.

Understanding Square Roots in Radical Form

The radical form of the square root of 29 is expressed as √29. This indicates that the number 29 is the radicand, and the radical symbol signifies that we are looking for the square root of 29.

Why Can't the Square Root of 29 Be Simplified?

The square root of 29 cannot be simplified because 29 is not a perfect square. A perfect square is a number that can be expressed as the product of an integer with itself. For example, 36 is a perfect square because it is 6 multiplied by 6. The square root of 36 can be simplified as √36 = √(6*6) = 6.

However, 29 is not a product of an integer with itself. Therefore, the square root of 29 cannot be simplified further than √29.

Calculating the Square Root of 29

The actual value of the square root of 29 can be calculated using a calculator or a computer program. The approximate value is 5.3851648. However, this is an irrational number, and its decimal representation goes on indefinitely without repeating.

Importance of Square Roots in Radical Form

Understanding square roots in radical form is crucial for advanced mathematical calculations, particularly in algebra, geometry, and trigonometry. It allows us to represent and work with numbers that do not have a whole number as their square root.

In various mathematical problems, we often need to simplify or manipulate square roots in radical form. For instance, when working with quadratic equations, we might need to express the solution in radical form to convey that the number is an irrational value.

Practical Applications of Square Roots

Square roots have numerous practical applications in various fields, including:

• Physics and Engineering: Square roots are used to calculate distances, velocities, and accelerations.
• Computer Science: Square roots are used in algorithms for tasks such as graphics rendering and scientific simulations.
• Statistics: Square roots are used in statistical analysis to calculate standard deviations and variance.
• Finance: Square roots are used in financial modeling to calculate volatility and risk.

Calculating the Square Root of 29 Using a Calculator

To calculate the square root of 29 using a calculator, follow these steps:

1. Enter the number 29 into the calculator.
2. Press the square root button (√).
3. The calculator will display the approximate value of the square root of 29.

Keep in mind that the actual value of the square root of 29 is an irrational number, and the calculator will provide an approximate value.

Conclusion

The square root of 29 in radical form is expressed as √29. This indicates that the number 29 is the radicand, and the radical symbol signifies that we are looking for the square root of 29. The square root of 29 cannot be simplified further than √29 because 29 is not a perfect square.

Understanding square roots in radical form is essential for advanced mathematical calculations and has numerous practical applications in various fields. By recognizing the importance of square roots in radical form, we can better appreciate the complexity and beauty of mathematics.