Square Root Of 108 In Radical Form Simplified

Quick Links :

Understanding Square Roots and Radical Form

Square roots and radical form are fundamental concepts in mathematics, particularly in algebra and geometry. A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16. However, not all numbers have a perfect square root, which is where radical form comes in.

What is Radical Form?

Radical form is a way of expressing a square root or other roots of a number in a simplified form. It involves using the radical symbol (√) followed by the number inside the radical. For instance, the square root of 2 can be written as √2. Radical form is particularly useful when dealing with numbers that do not have a perfect square root.

Simplifying Square Roots in Radical Form

Simplifying square roots in radical form involves finding the largest perfect square factor of the number inside the radical. This process helps to reduce the radical to its simplest form. For example, the square root of 12 can be simplified as √(4*3), which further simplifies to 2√3, because 4 is the largest perfect square factor of 12.

How to Simplify Square Roots in Radical Form

1. Find the largest perfect square factor: Identify the largest perfect square that divides the number inside the radical.
2. Break down the number: Express the number as a product of the perfect square factor and another number.
3. Simplify the radical: Take the square root of the perfect square factor and multiply it by the remaining number inside the radical.

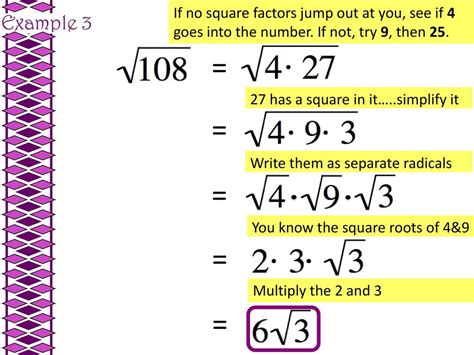
Calculating the Square Root of 108 in Radical Form

To calculate the square root of 108 in radical form, we follow the steps outlined above.

1. Find the largest perfect square factor: The largest perfect square factor of 108 is 36, because 36 is a perfect square (6*6) and divides 108.
2. Break down the number: Express 108 as 36*3, since 108 divided by 36 equals 3.
3. Simplify the radical: Take the square root of 36, which is 6, and multiply it by the square root of 3.

Therefore, the square root of 108 in radical form is 6√3.

Applications of Square Roots and Radical Form

Square roots and radical form have numerous applications in mathematics, science, and engineering. Some of these applications include:

• Geometry: Square roots are used to calculate distances, lengths, and areas in geometric shapes.
• Algebra: Radical form is essential in solving equations and inequalities involving square roots.
• Physics: Square roots are used to calculate velocities, accelerations, and energies in physics problems.
• Engineering: Radical form is applied in designing and optimizing systems, such as electronic circuits and mechanical systems.

Conclusion

In conclusion, understanding square roots and radical form is crucial in mathematics and its applications. Simplifying square roots in radical form involves finding the largest perfect square factor and breaking down the number inside the radical. The square root of 108 in radical form is 6√3, which demonstrates the application of these concepts.

We hope this article has been informative and helpful in your understanding of square roots and radical form. If you have any questions or need further clarification, please don't hesitate to ask.