5 Ways To Master Slope Intercept Form Practice

Quick Links :

Mastering slope-intercept form is a crucial milestone in algebra, enabling students to solve a wide range of linear equations and graph lines with ease. Slope-intercept form, denoted by y = mx + b, provides a straightforward way to describe the relationship between the x and y coordinates of a line. However, it requires consistent practice to fully grasp and apply this concept effectively.

The benefits of mastering slope-intercept form are numerous. It not only simplifies the process of graphing lines but also facilitates the identification of key characteristics such as the slope and y-intercept. By becoming proficient in this area, students can tackle more complex algebraic problems and develop a solid foundation for advanced math studies.

Despite its importance, mastering slope-intercept form can be a daunting task for many students. With the right approach and sufficient practice, however, anyone can become proficient in this area. Here are five ways to master slope-intercept form practice:

Understanding the Slope-Intercept Form Formula

The slope-intercept form formula, y = mx + b, is composed of three primary elements: m, x, and b. The slope, denoted by m, represents the rate at which the line rises or falls. The x value represents the input or independent variable, while the b value represents the y-intercept or the point at which the line intersects the y-axis.

To truly master slope-intercept form, it's essential to understand the relationship between these elements and how they affect the graph of the line. By recognizing the role of each component, students can begin to visualize the line and make predictions about its behavior.

Identifying the Slope and Y-Intercept

Identifying the slope and y-intercept is a critical step in mastering slope-intercept form. The slope can be calculated using the formula m = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are two points on the line. The y-intercept, on the other hand, can be determined by substituting the value of x into the equation.

For example, given the equation y = 2x + 3, the slope is 2, and the y-intercept is 3. This information can be used to graph the line and make predictions about its behavior.

Graphing Lines in Slope-Intercept Form

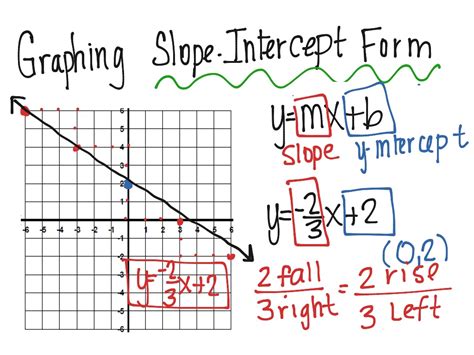
Graphing lines in slope-intercept form is a fundamental skill that requires practice to master. To graph a line, start by plotting the y-intercept, then use the slope to determine the next point on the line. By repeating this process, you can create a graph that accurately represents the equation.

For instance, given the equation y = -3x + 2, start by plotting the y-intercept at (0, 2). Then, use the slope to determine the next point on the line. Since the slope is -3, move down 3 units and right 1 unit to find the next point. Continue this process to create a graph that represents the equation.

Writing Equations in Slope-Intercept Form

Writing equations in slope-intercept form is another essential skill that requires practice to master. To write an equation in slope-intercept form, start by identifying the slope and y-intercept. Then, use the formula y = mx + b to create an equation that represents the line.

For example, given a line with a slope of 4 and a y-intercept of -2, the equation in slope-intercept form would be y = 4x - 2. By practicing this skill, students can become proficient in writing equations that accurately represent lines.

Practicing with Real-World Applications

Practicing with real-world applications is an effective way to master slope-intercept form. By applying the concept to real-world scenarios, students can develop a deeper understanding of the material and see its relevance in everyday life.

For instance, consider a scenario where a company's profit is directly proportional to the number of units sold. By using slope-intercept form, you can create an equation that represents the relationship between the profit and the number of units sold. This type of application can help students see the value in mastering slope-intercept form.

Using Online Resources and Practice Tests

Using online resources and practice tests is an excellent way to master slope-intercept form. There are numerous online resources available that provide practice problems, video tutorials, and interactive exercises to help students develop their skills.

Some popular online resources include Khan Academy, Mathway, and IXL. These websites offer a wide range of practice problems and exercises that cater to different skill levels and learning styles.

Mastering Slope-Intercept Form Takes Time and Practice

Mastering slope-intercept form takes time and practice. It's essential to be patient and persistent when working through practice problems and exercises. With consistent effort, students can develop a deep understanding of the material and become proficient in slope-intercept form.

Remember, mastering slope-intercept form is a journey, and it's essential to enjoy the process. By incorporating the strategies outlined above, students can develop a strong foundation in algebra and set themselves up for success in advanced math studies.

We hope you found this article helpful in your journey to master slope-intercept form. What strategies have you found most effective in practicing slope-intercept form? Share your thoughts and comments below!