Unlock The Ellipse Equation In 5 Simple Steps

Quick Links :

The ellipse equation - a fundamental concept in mathematics that can seem daunting at first, but fear not, dear reader, for we're about to break it down into 5 simple steps. Whether you're a student, teacher, or simply a math enthusiast, this article will guide you through the process of understanding and working with the ellipse equation.

In today's world, where precision and accuracy are crucial in various fields such as engineering, architecture, and physics, the ellipse equation plays a vital role. It's used to describe the shape of orbits, mirrors, and lenses, among other things. So, let's dive into the world of ellipses and unlock the secrets of the ellipse equation.

What is an Ellipse?

An ellipse is a closed curve on a plane surrounding two focal points such that the sum of the distances to the two focal points is constant. This definition might seem complex, but think of it like this: imagine a circle, but instead of being perfectly round, it's slightly flattened. That's basically an ellipse.

Understanding the Ellipse Equation

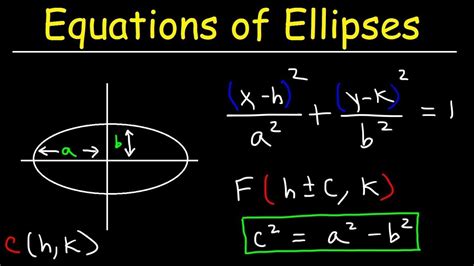
The ellipse equation is a mathematical formula that describes the shape of an ellipse. It's a quadratic equation in two variables, usually x and y. The general form of the ellipse equation is:

(x^2/a^2) + (y^2/b^2) = 1

where a and b are the lengths of the semi-major and semi-minor axes, respectively.

Breaking Down the Ellipse Equation

• The x^2/a^2 term represents the horizontal axis of the ellipse, while the y^2/b^2 term represents the vertical axis.
• The constants a and b determine the shape and size of the ellipse.
• The equation is equal to 1, which means that the sum of the squares of the distances from any point on the ellipse to the two focal points is constant.

Step 1: Identify the Center of the Ellipse

The first step in working with the ellipse equation is to identify the center of the ellipse. This is usually denoted by the coordinates (h, k). The center of the ellipse is the midpoint of the major axis.

How to Find the Center of the Ellipse

• If you're given the equation of the ellipse, you can find the center by looking at the coefficients of the x and y terms.
• If you're given the graph of the ellipse, you can find the center by looking at the midpoint of the major axis.

Step 2: Determine the Lengths of the Semi-Major and Semi-Minor Axes

The next step is to determine the lengths of the semi-major and semi-minor axes. These are usually denoted by the constants a and b, respectively.

How to Find the Lengths of the Semi-Major and Semi-Minor Axes

• If you're given the equation of the ellipse, you can find the lengths of the semi-major and semi-minor axes by looking at the coefficients of the x and y terms.
• If you're given the graph of the ellipse, you can find the lengths of the semi-major and semi-minor axes by measuring the distances from the center to the vertices.

Step 3: Write the Equation of the Ellipse

Now that you have the center and the lengths of the semi-major and semi-minor axes, you can write the equation of the ellipse.

How to Write the Equation of the Ellipse

• Use the general form of the ellipse equation: (x^2/a^2) + (y^2/b^2) = 1
• Plug in the values of the center, semi-major axis, and semi-minor axis.

Step 4: Graph the Ellipse

The next step is to graph the ellipse. This can be done using a variety of methods, including graphing calculators and computer software.

How to Graph the Ellipse

• Use a graphing calculator or computer software to graph the ellipse.
• Plot the center and vertices of the ellipse.
• Draw the major and minor axes.

Step 5: Solve Problems Involving the Ellipse Equation

The final step is to solve problems involving the ellipse equation. This can include finding the area of the ellipse, the perimeter of the ellipse, and the equation of the tangent line to the ellipse.

How to Solve Problems Involving the Ellipse Equation

• Use the equation of the ellipse to solve problems.
• Plug in the values of the center, semi-major axis, and semi-minor axis.
• Use algebraic techniques to solve the equation.

By following these 5 simple steps, you can unlock the secrets of the ellipse equation and become proficient in working with ellipses.

We hope this article has been informative and helpful. If you have any questions or comments, please don't hesitate to ask. Share this article with your friends and classmates, and don't forget to check out our other articles on mathematics and science.