Six Hundredths In Decimal Form Explained

Quick Links :

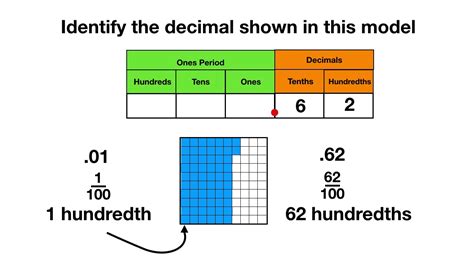
Converting fractions to decimals is an essential skill in mathematics, and it's a concept that many students struggle with. In this article, we'll focus on understanding six hundredths in decimal form, exploring its representation, conversion, and practical applications.

Understanding Fractions and Decimals

Before diving into six hundredths, it's crucial to understand the basics of fractions and decimals. A fraction represents a part of a whole, consisting of a numerator (the top number) and a denominator (the bottom number). Decimals, on the other hand, are a way of expressing fractions in a different form, using a point (.) to separate the whole number from the fractional part.

What is Six Hundredths?

Six hundredths is a fraction that represents six equal parts out of a total of 100. It's written as 6/100 and can be simplified to 3/50. However, when converting this fraction to a decimal, we need to divide the numerator (6) by the denominator (100).

Converting Six Hundredths to Decimal Form

To convert six hundredths to a decimal, we'll perform the division:

6 ÷ 100 = 0.06

So, six hundredths in decimal form is equal to 0.06.

Practical Applications of Six Hundredths

Understanding six hundredths in decimal form has various practical applications in everyday life:

• Finance: When dealing with interest rates or percentages, six hundredths can be used to represent small changes in value. For example, an interest rate of 0.06% might seem insignificant, but it can add up over time.
• Measurement: In measurement, six hundredths can represent a small fraction of a unit. For instance, in cooking, a recipe might require 0.06 ounces of a specific ingredient.
• Science: In scientific calculations, six hundredths can be used to represent small changes in data or experimental results.

Comparing Six Hundredths to Other Fractions

To better understand six hundredths, let's compare it to other fractions:

• Three hundredths: 3/100 = 0.03 (smaller than six hundredths)
• Nine hundredths: 9/100 = 0.09 (larger than six hundredths)
• One tenth: 1/10 = 0.1 (larger than six hundredths)

Key Differences and Similarities

When comparing these fractions, we notice that:

• Six hundredths is smaller than nine hundredths but larger than three hundredths.
• One tenth is larger than six hundredths, but they're both relatively small fractions.

Real-World Examples of Six Hundredths

Here are some real-world examples that illustrate the use of six hundredths:

• Bank interest: A savings account might offer an interest rate of 0.06% per annum, which can add up to a significant amount over time.
• Recipe ingredient: A recipe for a cake might require 0.06 ounces of salt, which is a small fraction of the total ingredients.
• Scientific measurement: A scientist might measure the length of an object to be 0.06 meters, which is a precise measurement.

Challenges and Misconceptions

When dealing with six hundredths, some common challenges and misconceptions include:

• Rounding errors: Rounding six hundredths to the nearest hundredth or tenth can lead to errors in calculations.
• Misunderstanding decimal representation: Some students might struggle to understand that 0.06 represents six hundredths, rather than six tenths.

Overcoming Challenges and Misconceptions

To overcome these challenges, it's essential to:

• Practice converting fractions to decimals and vice versa.
• Use real-world examples to illustrate the concept of six hundredths.
• Emphasize the importance of precision when working with decimals.

Conclusion

In conclusion, understanding six hundredths in decimal form is a crucial concept in mathematics, with various practical applications in finance, measurement, and science. By recognizing the importance of precision and using real-world examples, we can overcome challenges and misconceptions associated with this concept.

If you have any questions or comments about six hundredths or decimal representation, please share them below. We'd love to hear from you and provide further clarification on this topic.