4 Steps To Simplify Radicals Like 40

Quick Links :

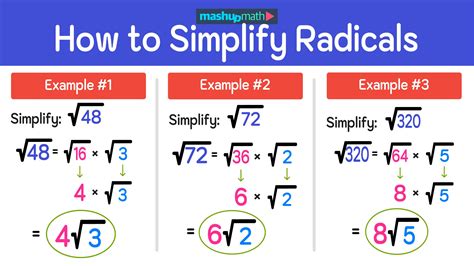
Radicals can be intimidating, especially when they involve large numbers like 40. However, simplifying radicals can be a straightforward process if you follow the right steps. In this article, we'll break down the process into four easy-to-follow steps, and provide examples and explanations to help you understand each step.

Step 1: Find the Largest Perfect Square Factor

The first step in simplifying a radical is to find the largest perfect square factor of the number inside the radical. A perfect square is a number that can be expressed as the square of an integer, such as 4, 9, or 16. To find the largest perfect square factor, you can start by factoring the number inside the radical into its prime factors.

For example, let's simplify the radical √40. The prime factorization of 40 is:

40 = 2 × 2 × 2 × 5

Now, look for the largest perfect square factor. In this case, it's 4, which is the square of 2.

How to Find the Largest Perfect Square Factor

To find the largest perfect square factor, follow these steps:

• Factor the number inside the radical into its prime factors.
• Look for pairs of identical prime factors.
• Combine the pairs to form a perfect square.

In the case of √40, we found that the largest perfect square factor is 4, which is the square of 2.

Step 2: Write the Radical as a Product

Once you've found the largest perfect square factor, you can write the radical as a product of the perfect square and a remaining factor. In the case of √40, we can write:

√40 = √(4 × 10)

Now, we can simplify the radical by taking the square root of the perfect square factor, which is 2.

How to Write the Radical as a Product

To write the radical as a product, follow these steps:

• Identify the largest perfect square factor.
• Divide the number inside the radical by the perfect square factor.
• Write the radical as a product of the perfect square and the remaining factor.

In the case of √40, we wrote the radical as a product of the perfect square 4 and the remaining factor 10.

Step 3: Simplify the Radical

Now that we've written the radical as a product, we can simplify it by taking the square root of the perfect square factor. In the case of √40, we have:

√40 = √(4 × 10) = √4 × √10 = 2√10

And that's the simplified form of the radical!

How to Simplify the Radical

To simplify the radical, follow these steps:

• Take the square root of the perfect square factor.
• Write the remaining factor inside the radical.

In the case of √40, we took the square root of the perfect square factor 4, which is 2, and wrote the remaining factor 10 inside the radical.

Step 4: Check for Further Simplification

Finally, we need to check if the radical can be simplified further. In the case of 2√10, there are no more perfect square factors, so we can't simplify it further.

However, in some cases, you may find that the remaining factor can be simplified further. For example, if we had √(16 × 5), we could simplify it further by taking the square root of the perfect square factor 16, which is 4.

How to Check for Further Simplification

To check for further simplification, follow these steps:

• Look for any remaining perfect square factors.
• If you find any, simplify them further.
• If not, the radical is fully simplified.

In the case of 2√10, we couldn't find any remaining perfect square factors, so we concluded that the radical is fully simplified.

By following these four steps, you can simplify radicals like √40 with ease. Remember to find the largest perfect square factor, write the radical as a product, simplify the radical, and check for further simplification.

Now, we'd like to hear from you! Do you have any questions or examples you'd like to share? Please leave a comment below, and we'll do our best to help.