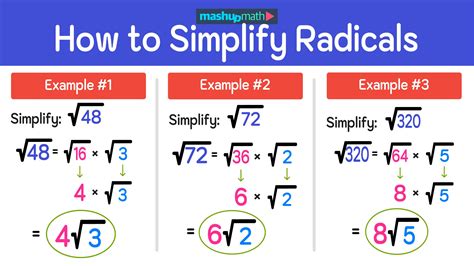
Radicals can be a challenging concept in mathematics, but simplifying them doesn't have to be a daunting task. In this article, we will explore the concept of radicals, the importance of simplifying them, and provide a step-by-step guide on how to simplify radicals in just one step.
What are Radicals?

Radicals are mathematical expressions that contain a root symbol (√). They are used to represent the root of a number or an expression. For example, √16 is the square root of 16, which equals 4. Radicals can be simplified by finding the factors of the number inside the radical and expressing it in the simplest form possible.
Why Simplify Radicals?

Simplifying radicals is essential in mathematics because it helps to:
- Make calculations easier and more manageable
- Reduce errors by avoiding complicated expressions
- Enhance understanding of mathematical concepts
- Improve problem-solving skills
Simplifying Radicals in 1 Step

To simplify radicals in just one step, follow these steps:
- Find the largest perfect square: Identify the largest perfect square that is a factor of the number inside the radical.
- Write the number as a product: Express the number inside the radical as a product of the perfect square and another factor.
- Simplify the radical: Simplify the radical by taking the square root of the perfect square and writing it outside the radical.
Example: Simplify √162
To simplify √162, follow the steps above:
- Find the largest perfect square: 81 (since 81 is a perfect square and a factor of 162)
- Write the number as a product: 162 = 81 × 2
- Simplify the radical: √162 = √(81 × 2) = √81 × √2 = 9√2
Therefore, the simplest form of √162 is 9√2.
Common Mistakes to Avoid

When simplifying radicals, it's essential to avoid common mistakes such as:
- Not finding the largest perfect square factor
- Not expressing the number as a product correctly
- Not simplifying the radical correctly
Practical Applications of Simplifying Radicals

Simplifying radicals has practical applications in various fields, including:
- Physics: Simplifying radicals is crucial in calculating distances, velocities, and accelerations.
- Engineering: Simplifying radicals is essential in designing and optimizing systems.
- Computer Science: Simplifying radicals is used in algorithms and data analysis.
Conclusion
Simplifying radicals is a crucial skill in mathematics that can be achieved in just one step. By following the steps outlined in this article, you can simplify radicals with ease and confidence. Remember to find the largest perfect square factor, write the number as a product, and simplify the radical. Avoid common mistakes and apply simplifying radicals to practical problems in physics, engineering, and computer science.Share your thoughts!
Have you struggled with simplifying radicals in the past? Share your experiences and tips in the comments below. Don't forget to share this article with your friends and classmates who may benefit from learning how to simplify radicals in just one step.
FAQ Section
What is the purpose of simplifying radicals?
+The purpose of simplifying radicals is to make calculations easier and more manageable, reduce errors, and enhance understanding of mathematical concepts.
How do I simplify radicals in one step?
+To simplify radicals in one step, find the largest perfect square factor, write the number as a product, and simplify the radical.
What are some common mistakes to avoid when simplifying radicals?
+Common mistakes to avoid when simplifying radicals include not finding the largest perfect square factor, not expressing the number as a product correctly, and not simplifying the radical correctly.