Master Vertex Form With 10 Essential Practice Exercises

Quick Links :

Mastering the vertex form of a quadratic equation is a crucial skill for any student of mathematics. The vertex form, also known as the "a" form, is a way of expressing a quadratic equation in a more convenient and useful way. In this article, we will explore the vertex form, its benefits, and provide 10 essential practice exercises to help you master it.

What is Vertex Form?

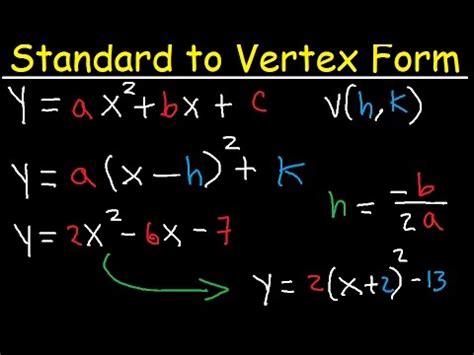
The vertex form of a quadratic equation is given by:

y = a(x - h)^2 + k

where (h, k) is the vertex of the parabola, and "a" is the coefficient that determines the direction and width of the parabola. This form is particularly useful for finding the vertex, axis of symmetry, and x-intercepts of a quadratic equation.

Benefits of Vertex Form

The vertex form has several benefits that make it a popular choice among mathematicians and students. Some of the benefits include:

• Easy identification of the vertex and axis of symmetry
• Simplified process for finding x-intercepts
• Useful for graphing and analyzing quadratic equations
• Convenient for solving quadratic equations

How to Convert to Vertex Form

To convert a quadratic equation to vertex form, you can follow these steps:

1. Complete the square by adding and subtracting the square of half the coefficient of x.
2. Factor the perfect square trinomial.
3. Write the equation in vertex form.

For example, let's convert the quadratic equation x^2 + 6x + 8 = 0 to vertex form:

x^2 + 6x + 8 = 0 x^2 + 6x + 9 - 1 = 0 (x + 3)^2 - 1 = 0 x + 3 = ±1 x = -3 ± 1 x = -2, -4

The vertex form of the equation is:

y = (x + 3)^2 - 1

Practice Exercises

Now that you know how to convert to vertex form, it's time to practice! Here are 10 essential practice exercises to help you master the vertex form:

1. Convert the quadratic equation x^2 + 4x + 4 = 0 to vertex form.
2. Find the vertex and axis of symmetry of the quadratic equation x^2 - 2x - 3 = 0.
3. Convert the quadratic equation x^2 - 6x + 8 = 0 to vertex form.
4. Find the x-intercepts of the quadratic equation x^2 + 2x - 6 = 0.
5. Convert the quadratic equation x^2 + 8x + 16 = 0 to vertex form.
6. Find the vertex and axis of symmetry of the quadratic equation x^2 + 4x - 5 = 0.
7. Convert the quadratic equation x^2 - 8x + 16 = 0 to vertex form.
8. Find the x-intercepts of the quadratic equation x^2 - 2x - 8 = 0.
9. Convert the quadratic equation x^2 + 10x + 25 = 0 to vertex form.
10. Find the vertex and axis of symmetry of the quadratic equation x^2 - 10x + 24 = 0.

Solutions to Practice Exercises

Here are the solutions to the practice exercises:

1. y = (x + 2)^2
2. Vertex: (-1, -4), Axis of Symmetry: x = -1
3. y = (x - 3)^2 - 1
4. x = -3, 2
5. y = (x + 4)^2
6. Vertex: (-2, 9), Axis of Symmetry: x = -2
7. y = (x - 4)^2
8. x = -2, 4
9. y = (x + 5)^2
10. Vertex: (5, -1), Axis of Symmetry: x = 5

Conclusion

Mastering the vertex form of a quadratic equation takes practice and patience. With these 10 essential practice exercises, you'll be well on your way to becoming a pro at converting quadratic equations to vertex form. Remember to take your time, follow the steps, and practice regularly to reinforce your understanding.

We hope this article has been helpful in your journey to master the vertex form. If you have any questions or need further clarification, please don't hesitate to ask. Happy learning!