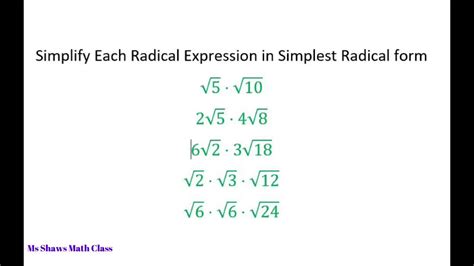
Radicals, also known as roots, are a fundamental concept in mathematics, particularly in algebra and geometry. They can be intimidating at first, but simplifying radicals can be made easy with a little practice and understanding of the rules. In this article, we will delve into the world of radicals, explore the concept of simplest form, and provide examples to help you master this skill.
Radicals are used to represent numbers that cannot be expressed as simple fractions or whole numbers. They are denoted by a symbol called the radical sign, which looks like a checkmark (√). The number inside the radical sign is called the radicand. For example, in the expression √16, 16 is the radicand.
Why Simplify Radicals?

Simplifying radicals is essential in mathematics because it helps to:
- Reduce complex expressions to simpler forms
- Make calculations easier and faster
- Avoid confusion when working with radicals
- Improve understanding of mathematical concepts
Rules for Simplifying Radicals

To simplify radicals, you need to follow these basic rules:
- Look for perfect squares: If the radicand is a perfect square, you can simplify the radical by finding the square root of the radicand. For example, √16 = √(4 × 4) = 4.
- Factor out perfect squares: If the radicand is not a perfect square, look for perfect squares that can be factored out. For example, √48 = √(16 × 3) = √16 × √3 = 4√3.
- Use the product rule: The product rule states that √(ab) = √a × √b. For example, √(4 × 9) = √4 × √9 = 2 × 3 = 6.
- Use the quotient rule: The quotient rule states that √(a/b) = √a / √b. For example, √(16/4) = √16 / √4 = 4/2 = 2.
Examples of Simplifying Radicals
Let's practice simplifying radicals with some examples:
- Simplify √25.
Answer: √25 = √(5 × 5) = 5
- Simplify √48.
Answer: √48 = √(16 × 3) = √16 × √3 = 4√3
- Simplify √(4 × 9).
Answer: √(4 × 9) = √4 × √9 = 2 × 3 = 6
- Simplify √(16/4).
Answer: √(16/4) = √16 / √4 = 4/2 = 2
Simplest Form Examples

Simplest form refers to the simplest way to express a radical. Here are some examples:
- √16 = 4 ( simplest form)
- √48 = 4√3 (simplest form)
- √(4 × 9) = 6 (simplest form)
- √(16/4) = 2 (simplest form)
More Examples of Simplest Form
Let's practice finding the simplest form of radicals with some more examples:
- Simplify √75.
Answer: √75 = √(25 × 3) = √25 × √3 = 5√3 (simplest form)
- Simplify √(9 × 16).
Answer: √(9 × 16) = √9 × √16 = 3 × 4 = 12 (simplest form)
- Simplify √(25/5).
Answer: √(25/5) = √25 / √5 = 5/√5 (simplest form)
Conclusion: Mastering Simplifying Radicals

Simplifying radicals is a crucial skill in mathematics, and with practice and understanding of the rules, you can master it. Remember to look for perfect squares, factor out perfect squares, and use the product and quotient rules to simplify radicals. By following these rules and practicing with examples, you can simplify radicals with ease and become proficient in mathematics.
FAQ Section:
What is the purpose of simplifying radicals?
+Simplifying radicals helps to reduce complex expressions to simpler forms, making calculations easier and faster.
What are the basic rules for simplifying radicals?
+The basic rules for simplifying radicals are: look for perfect squares, factor out perfect squares, use the product rule, and use the quotient rule.
What is the simplest form of a radical?
+The simplest form of a radical is the simplest way to express a radical, which is achieved by following the basic rules of simplifying radicals.