3 Simple Ways To Simplify A Fraction To 12

Quick Links :

Reducing fractions to their simplest form is an essential skill in mathematics, and it's used in various everyday applications. When it comes to simplifying fractions to a denominator of 12, it's crucial to understand the concept of equivalent fractions and how to manipulate them. In this article, we will explore three simple ways to simplify a fraction to a denominator of 12.

Understanding Equivalent Fractions

Before diving into the methods, it's essential to grasp the concept of equivalent fractions. Equivalent fractions are fractions that have the same value, but with different numerators and denominators. For example, 1/2 is equivalent to 2/4, 3/6, and 6/12. To simplify a fraction to a denominator of 12, we need to find an equivalent fraction that has 12 as its denominator.

Method 1: Multiplying by a Whole Number

One way to simplify a fraction to a denominator of 12 is to multiply both the numerator and denominator by a whole number. This method is based on the concept of equivalent fractions. By multiplying both the numerator and denominator by the same whole number, we can maintain the value of the fraction while changing its denominator.

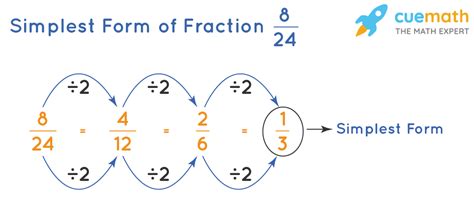
For example, let's simplify the fraction 1/3 to a denominator of 12. We can multiply both the numerator and denominator by 4, which gives us:

1/3 = (1 × 4) / (3 × 4) = 4/12

This method is straightforward and can be applied to any fraction. However, it requires us to find the correct whole number to multiply by, which can be time-consuming.

Method 2: Dividing by a Common Factor

Another way to simplify a fraction to a denominator of 12 is to divide both the numerator and denominator by a common factor. This method is based on the concept of simplifying fractions by canceling out common factors.

For example, let's simplify the fraction 6/12 to its simplest form. We can divide both the numerator and denominator by 2, which gives us:

6/12 = (6 ÷ 2) / (12 ÷ 2) = 3/6

We can further simplify the fraction by dividing both the numerator and denominator by 3, which gives us:

3/6 = (3 ÷ 3) / (6 ÷ 3) = 1/2

This method is efficient and can be used to simplify fractions quickly. However, it requires us to find the greatest common factor (GCF) of the numerator and denominator, which can be challenging.

Method 3: Using a Conversion Chart

A third way to simplify a fraction to a denominator of 12 is to use a conversion chart. A conversion chart is a table that lists equivalent fractions with different denominators. By using a conversion chart, we can quickly find the equivalent fraction with a denominator of 12.

For example, let's simplify the fraction 3/4 to a denominator of 12. We can use a conversion chart to find the equivalent fraction, which gives us:

3/4 = 9/12

This method is convenient and can be used to simplify fractions quickly. However, it requires us to have a conversion chart handy, which may not always be the case.

Conclusion and Next Steps

Simplifying fractions to a denominator of 12 is an essential skill in mathematics, and it can be achieved using three simple methods. By understanding equivalent fractions and using the methods outlined in this article, we can simplify fractions quickly and accurately.

To further develop your skills in simplifying fractions, practice using the methods outlined in this article. Start with simple fractions and gradually move on to more complex ones. You can also use online resources, such as fraction calculators and conversion charts, to help you simplify fractions.

We hope this article has been helpful in your journey to master simplifying fractions. If you have any questions or comments, please don't hesitate to share them with us.