7.003 In Standard Form Explained Simply

Quick Links :

The concept of numbers in standard form can be a bit tricky to grasp, especially for those who are new to mathematics. However, with a simple explanation, you'll be able to understand what 7.003 in standard form means and how to work with it.

Standard form is a way of expressing numbers in a concise and readable format. It's commonly used in science, engineering, and mathematics to represent very large or very small numbers. The standard form of a number is written in the form:

a × 10^n

where 'a' is a number between 1 and 10, and 'n' is an integer that represents the power of 10.

So, let's break down the number 7.003 in standard form.

What is 7.003 in Standard Form?

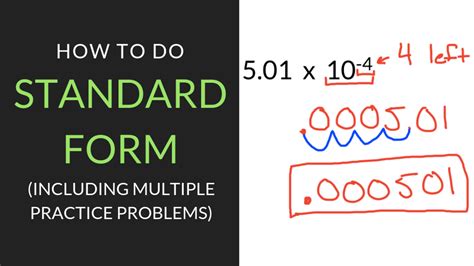
To express 7.003 in standard form, we need to move the decimal point to the left until we have a number between 1 and 10. In this case, we need to move the decimal point 3 places to the left, which gives us:

7.003 = 7.003 × 10^3

However, this is not the correct standard form. We need to make sure that the number before the multiplication sign is between 1 and 10. To do this, we can rewrite the number as:

7.003 = 7.003 × 10^3 = 7.003 × 10^3 × 10^(-3+3) = 7.003 × 10^0 = 7.003 × 1

Since 7.003 is already between 1 and 10, we can simply write it as:

7.003 = 7.003 × 10^0

Why is Standard Form Important?

Standard form is important in mathematics and science because it allows us to easily compare and manipulate very large or very small numbers. It's also useful for simplifying complex calculations and avoiding errors.

In science, standard form is used to express measurements such as the size of atoms, the distance between stars, and the speed of light. In engineering, it's used to design and build complex systems, such as bridges, buildings, and electronic circuits.

How to Convert Numbers to Standard Form

Converting numbers to standard form is a straightforward process. Here are the steps:

1. Move the decimal point to the left or right until you have a number between 1 and 10.
2. Count the number of places you moved the decimal point. This will give you the power of 10.
3. Write the number in standard form using the format: a × 10^n

For example, let's convert the number 456,789 to standard form.

1. Move the decimal point 5 places to the left: 456,789 = 4.56789
2. Count the number of places: 5
3. Write the number in standard form: 4.56789 × 10^5

Common Mistakes When Working with Standard Form

When working with standard form, there are a few common mistakes to watch out for:

• Moving the decimal point the wrong number of places
• Forgetting to include the power of 10
• Writing the number in the wrong format

To avoid these mistakes, make sure to double-check your work and use the correct format.

Real-World Applications of Standard Form

Standard form has many real-world applications in science, engineering, and mathematics. Here are a few examples:

• Measuring the size of atoms and molecules
• Calculating the distance between stars and galaxies
• Designing electronic circuits and microchips
• Building bridges and buildings
• Modeling population growth and disease spread

In conclusion, standard form is an important concept in mathematics and science that allows us to easily compare and manipulate very large or very small numbers. By understanding how to convert numbers to standard form and avoiding common mistakes, you'll be able to tackle complex problems with confidence.

Take Action!

Now that you've learned about standard form, it's time to put your knowledge into practice. Try converting some numbers to standard form and see how it can help you in your studies or work. Share your experiences and tips in the comments below, and don't forget to share this article with your friends and colleagues.