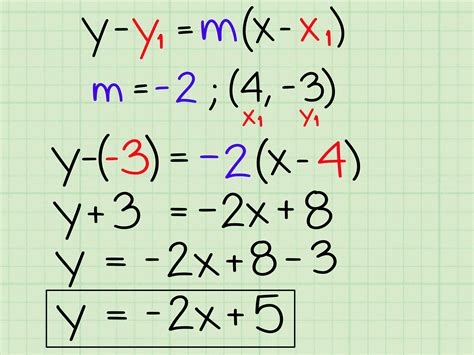When dealing with linear equations, one of the most useful forms is the slope-intercept form, which is y = mx + b, where m represents the slope of the line and b represents the y-intercept. This form makes it easy to identify the slope and y-intercept of a line, which is crucial in various mathematical and real-world applications. However, not all linear equations are given in slope-intercept form. Fortunately, there are several ways to rewrite an equation in slope-intercept form.

Why Rewrite Equations in Slope-Intercept Form?
Rewriting equations in slope-intercept form has several benefits. Firstly, it makes it easy to identify the slope and y-intercept of a line, which is crucial in graphing and analyzing linear equations. Secondly, it allows for easy comparison of different linear equations. Finally, it is a convenient form for solving systems of linear equations.
Method 1: Converting from Standard Form
One common way to rewrite an equation in slope-intercept form is to convert it from standard form, which is Ax + By = C. To convert from standard form to slope-intercept form, we need to isolate y.
For example, consider the equation 2x + 3y = 6. To convert this equation to slope-intercept form, we need to isolate y.
2x + 3y = 6
Subtract 2x from both sides:
3y = -2x + 6
Divide both sides by 3:
y = (-2/3)x + 2

Method 2: Converting from Point-Slope Form
Another way to rewrite an equation in slope-intercept form is to convert it from point-slope form, which is y - y1 = m(x - x1). To convert from point-slope form to slope-intercept form, we need to expand and simplify the equation.
For example, consider the equation y - 2 = 3(x - 1). To convert this equation to slope-intercept form, we need to expand and simplify.
y - 2 = 3(x - 1)
Expand the right-hand side:
y - 2 = 3x - 3
Add 2 to both sides:
y = 3x - 1

Method 3: Using the Slope Formula
If we are given two points on a line, we can use the slope formula to find the slope of the line, and then use the point-slope form to rewrite the equation in slope-intercept form.
For example, consider the points (2, 3) and (4, 5). To find the slope of the line, we can use the slope formula:
m = (y2 - y1) / (x2 - x1)
m = (5 - 3) / (4 - 2)
m = 2 / 2
m = 1
Now that we have the slope, we can use the point-slope form to rewrite the equation in slope-intercept form.
y - 3 = 1(x - 2)
Expand the right-hand side:
y - 3 = x - 2
Add 3 to both sides:
y = x + 1

Method 4: Graphing the Equation
If we are given a graph of a line, we can use the graph to find the slope and y-intercept of the line, and then rewrite the equation in slope-intercept form.
For example, consider the graph of a line that passes through the points (1, 2) and (3, 4). From the graph, we can see that the y-intercept is 1 and the slope is 1.
Now that we have the slope and y-intercept, we can rewrite the equation in slope-intercept form.
y = mx + b
y = x + 1

Method 5: Using a Table of Values
If we are given a table of values for a linear equation, we can use the table to find the slope and y-intercept of the line, and then rewrite the equation in slope-intercept form.
For example, consider the table of values below:
| x | y |
|---|---|
| 1 | 2 |
| 2 | 4 |
| 3 | 6 |
From the table, we can see that the slope is 2 and the y-intercept is 0.
Now that we have the slope and y-intercept, we can rewrite the equation in slope-intercept form.
y = mx + b
y = 2x

In conclusion, there are several ways to rewrite an equation in slope-intercept form, including converting from standard form, point-slope form, using the slope formula, graphing the equation, and using a table of values. Each method has its own advantages and disadvantages, and the choice of method depends on the specific problem and the information given.
We hope this article has been informative and helpful in explaining the different methods of rewriting equations in slope-intercept form. If you have any questions or need further clarification, please don't hesitate to ask. Share this article with your friends and classmates who may also benefit from it.
What is slope-intercept form?
+Slope-intercept form is a way of writing a linear equation in the form y = mx + b, where m is the slope of the line and b is the y-intercept.
Why is it important to rewrite equations in slope-intercept form?
+Rewriting equations in slope-intercept form makes it easy to identify the slope and y-intercept of a line, which is crucial in graphing and analyzing linear equations.
What are the different methods of rewriting equations in slope-intercept form?
+The different methods of rewriting equations in slope-intercept form include converting from standard form, point-slope form, using the slope formula, graphing the equation, and using a table of values.