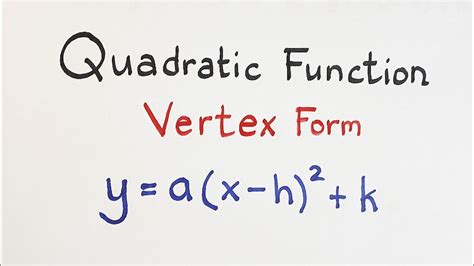
Quadratic functions are a fundamental concept in algebra, and being able to rewrite them in standard form is an essential skill for any math student. In this article, we will explore five different methods for rewriting quadratic functions in standard form, including completing the square, using the quadratic formula, and more.
What is Standard Form?

Before we dive into the different methods for rewriting quadratic functions in standard form, let's first define what we mean by standard form. The standard form of a quadratic function is ax^2 + bx + c, where a, b, and c are constants, and x is the variable. This form is useful because it allows us to easily identify the vertex of the parabola, as well as the axis of symmetry.
Method 1: Completing the Square

One of the most common methods for rewriting quadratic functions in standard form is completing the square. This method involves adding and subtracting a constant term to create a perfect square trinomial. Here's an example of how to complete the square:
x^2 + 6x + 8 =?
To complete the square, we first need to add and subtract (b/2)^2, where b is the coefficient of the x term. In this case, b = 6, so we add and subtract (6/2)^2 = 9.
x^2 + 6x + 9 - 9 + 8 =?
Next, we can factor the perfect square trinomial:
(x + 3)^2 - 1 =?
So, the quadratic function x^2 + 6x + 8 can be rewritten in standard form as (x + 3)^2 - 1.
Example: Rewrite the quadratic function x^2 + 4x + 3 in standard form using the completing the square method.
To complete the square, we first need to add and subtract (b/2)^2, where b is the coefficient of the x term. In this case, b = 4, so we add and subtract (4/2)^2 = 4.
x^2 + 4x + 4 - 4 + 3 =?
Next, we can factor the perfect square trinomial:
(x + 2)^2 - 1 =?
So, the quadratic function x^2 + 4x + 3 can be rewritten in standard form as (x + 2)^2 - 1.
Method 2: Using the Quadratic Formula

Another method for rewriting quadratic functions in standard form is to use the quadratic formula. The quadratic formula is x = (-b ± √(b^2 - 4ac)) / 2a, where a, b, and c are the coefficients of the quadratic function.
To use the quadratic formula to rewrite a quadratic function in standard form, we first need to identify the values of a, b, and c. Then, we can plug these values into the quadratic formula and simplify.
Example: Rewrite the quadratic function x^2 + 5x + 6 in standard form using the quadratic formula.
To use the quadratic formula, we first need to identify the values of a, b, and c. In this case, a = 1, b = 5, and c = 6.
x = (-5 ± √(5^2 - 4(1)(6))) / 2(1)
x = (-5 ± √(25 - 24)) / 2
x = (-5 ± √1) / 2
x = (-5 ± 1) / 2
x = (-5 + 1) / 2 or x = (-5 - 1) / 2
x = -2 or x = -3
So, the quadratic function x^2 + 5x + 6 can be rewritten in standard form as (x + 2)(x + 3).
Method 3: Factoring

Factoring is another method for rewriting quadratic functions in standard form. This method involves finding two binomials whose product is equal to the quadratic function.
Example: Rewrite the quadratic function x^2 + 7x + 12 in standard form using factoring.
To factor the quadratic function, we need to find two numbers whose product is equal to 12 and whose sum is equal to 7. These numbers are 3 and 4, so we can write the quadratic function as:
x^2 + 7x + 12 = (x + 3)(x + 4)
So, the quadratic function x^2 + 7x + 12 can be rewritten in standard form as (x + 3)(x + 4).
Method 4: Using a Graphing Calculator

A graphing calculator can also be used to rewrite quadratic functions in standard form. This method involves graphing the quadratic function and then using the calculator to find the vertex and axis of symmetry.
Example: Rewrite the quadratic function x^2 + 3x + 2 in standard form using a graphing calculator.
To use a graphing calculator to rewrite the quadratic function, we first need to graph the function. Then, we can use the calculator to find the vertex and axis of symmetry.
Using the calculator, we find that the vertex is at (-1.5, -0.25) and the axis of symmetry is x = -1.5.
So, the quadratic function x^2 + 3x + 2 can be rewritten in standard form as (x + 1.5)^2 - 0.25.
Method 5: Using a Table of Values

A table of values can also be used to rewrite quadratic functions in standard form. This method involves creating a table of values for the quadratic function and then using the table to find the vertex and axis of symmetry.
Example: Rewrite the quadratic function x^2 + 2x + 1 in standard form using a table of values.
To use a table of values to rewrite the quadratic function, we first need to create a table of values for the function.
| x | y |
|---|---|
| -2 | 5 |
| -1 | 2 |
| 0 | 1 |
| 1 | 2 |
| 2 | 5 |
Using the table, we can see that the vertex is at (0, 1) and the axis of symmetry is x = 0.
So, the quadratic function x^2 + 2x + 1 can be rewritten in standard form as (x + 0)^2 + 1.
In conclusion, there are many different methods for rewriting quadratic functions in standard form. Each method has its own advantages and disadvantages, and the choice of method will depend on the specific problem and the tools available.
We hope this article has been helpful in explaining the different methods for rewriting quadratic functions in standard form. Do you have any questions or comments about this topic? If so, please let us know in the comments section below.
What is the standard form of a quadratic function?
+The standard form of a quadratic function is ax^2 + bx + c, where a, b, and c are constants, and x is the variable.
How do I complete the square to rewrite a quadratic function in standard form?
+To complete the square, add and subtract (b/2)^2 to the quadratic function, where b is the coefficient of the x term. Then, factor the perfect square trinomial.