Rewrite In Vertex Form Calculator Made Easy

Quick Links :

Understanding the concept of vertex form is essential in mathematics, particularly in algebra and geometry. It provides a unique way to express quadratic equations, revealing crucial information about the parabola they represent. However, converting a quadratic equation from standard form to vertex form can be challenging without the right tools. This is where a vertex form calculator comes in, making the process easier and more efficient. In this article, we will explore the concept of vertex form, its significance, and how a vertex form calculator can simplify the conversion process.

What is Vertex Form?

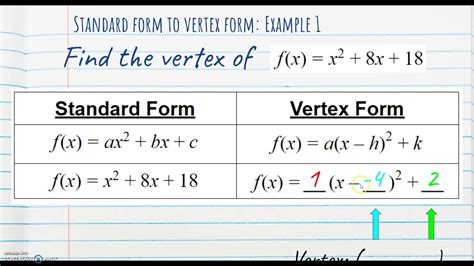
Vertex form, also known as the vertex representation, is a way to express a quadratic equation in the form f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. The vertex is the point where the parabola changes direction, and it is a critical point in understanding the shape and orientation of the parabola.

Benefits of Vertex Form

Vertex form offers several benefits over standard form:

• It provides a clear and concise way to express quadratic equations, making it easier to analyze and understand the parabola.
• It reveals the vertex of the parabola, which is essential in determining the maximum or minimum value of the function.
• It simplifies the process of finding the axis of symmetry, which is critical in graphing the parabola.

How to Convert to Vertex Form

Converting a quadratic equation from standard form to vertex form involves a series of steps. Here's a general outline:

1. Start with the standard form of the quadratic equation: f(x) = ax^2 + bx + c.
2. Complete the square by adding and subtracting (b/2)^2 to the equation.
3. Factor the perfect square trinomial to obtain the vertex form.

For example, consider the quadratic equation f(x) = x^2 + 6x + 8. To convert it to vertex form:

1. Add and subtract (6/2)^2 = 9 to the equation: f(x) = x^2 + 6x + 9 - 9 + 8.
2. Factor the perfect square trinomial: f(x) = (x + 3)^2 - 1.

The resulting equation is in vertex form, with (h, k) = (-3, -1).

Challenges in Converting to Vertex Form

While the process of converting to vertex form is straightforward, it can be challenging without the right tools. Some common difficulties include:

• Completing the square, which requires careful calculation and attention to detail.
• Factoring the perfect square trinomial, which can be time-consuming and prone to errors.
• Identifying the vertex, which requires a deep understanding of the parabola's shape and orientation.

How a Vertex Form Calculator Can Help

A vertex form calculator is a tool that simplifies the process of converting a quadratic equation from standard form to vertex form. It eliminates the need for manual calculations, reducing the risk of errors and saving time. With a vertex form calculator, you can:

• Enter the quadratic equation in standard form and obtain the vertex form instantly.
• Avoid the challenges of completing the square and factoring the perfect square trinomial.
• Easily identify the vertex and understand the parabola's shape and orientation.

Benefits of Using a Vertex Form Calculator

Using a vertex form calculator offers several benefits, including:

• Increased accuracy, as the calculator eliminates the risk of manual errors.
• Improved efficiency, as the calculator saves time and reduces the need for manual calculations.
• Enhanced understanding, as the calculator provides a clear and concise representation of the parabola.

Conclusion

In conclusion, vertex form is a powerful tool in mathematics, providing a unique way to express quadratic equations and reveal crucial information about the parabola. However, converting to vertex form can be challenging without the right tools. A vertex form calculator simplifies the process, eliminating the need for manual calculations and reducing the risk of errors. By using a vertex form calculator, you can improve your understanding of quadratic equations, increase your accuracy, and enhance your efficiency.

We hope this article has been informative and helpful. Please share your thoughts and feedback in the comments section below. If you have any questions or need further clarification, feel free to ask.