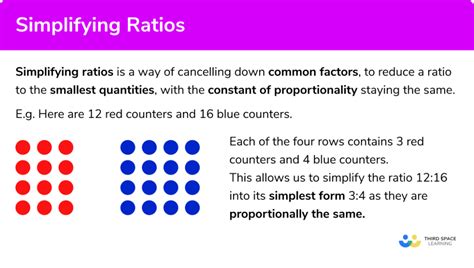
Ratios are a fundamental concept in mathematics, used to describe the relationship between two quantities. They are essential in various fields, including finance, science, and engineering. However, working with ratios can be complex, especially when dealing with multiple components or proportions. In this article, we will explore five ways to simplify ratios, making it easier to work with them and understand their implications.
Understanding Ratios
Before diving into simplification methods, it's essential to understand what ratios are and how they work. A ratio is a way of comparing two quantities, often denoted as a fraction (e.g., 2:3 or 2/3). The ratio represents the relationship between the two quantities, where the first value is the part and the second value is the whole.

Method 1: Simplifying by Greatest Common Divisor (GCD)
One of the most common methods to simplify ratios is by finding the greatest common divisor (GCD) of the two quantities. The GCD is the largest number that divides both quantities without leaving a remainder.
For example, let's simplify the ratio 12:18.
- Find the GCD of 12 and 18, which is 6.
- Divide both quantities by the GCD: 12 ÷ 6 = 2 and 18 ÷ 6 = 3.
- The simplified ratio is 2:3.

Benefits of GCD Simplification
- Simplifies ratios to their simplest form.
- Easy to calculate, especially for small quantities.
- Helps to identify equivalent ratios.
Method 2: Simplifying by Canceling Out Common Factors
Another method to simplify ratios is by canceling out common factors. This involves identifying the common factors between the two quantities and canceling them out.
For example, let's simplify the ratio 4:12.
- Identify the common factor: 4.
- Cancel out the common factor: 4/4 = 1 and 12/4 = 3.
- The simplified ratio is 1:3.

Benefits of Canceling Out Common Factors
- Quick and easy method for simplification.
- Helps to identify equivalent ratios.
- Useful for ratios with large quantities.
Method 3: Simplifying by Converting to Equivalent Ratios
Equivalent ratios are ratios that have the same value but with different quantities. By converting a ratio to its equivalent form, we can simplify it.
For example, let's simplify the ratio 3:9.
- Convert the ratio to its equivalent form: 1:3 (by dividing both quantities by 3).
- The simplified ratio is 1:3.

Benefits of Equivalent Ratios Simplification
- Helps to identify equivalent ratios.
- Useful for ratios with large quantities.
- Simplifies ratios to their simplest form.
Method 4: Simplifying by Using Proportional Reasoning
Proportional reasoning involves using the concept of proportionality to simplify ratios. This method is useful for ratios that involve proportions.
For example, let's simplify the ratio 2:4:6.
- Identify the proportion: 2:4 = 1:2 and 4:6 = 2:3.
- Use proportional reasoning to simplify the ratio: 1:2:3.
- The simplified ratio is 1:2:3.

Benefits of Proportional Reasoning Simplification
- Helps to identify proportions and equivalent ratios.
- Useful for ratios with multiple components.
- Simplifies ratios to their simplest form.
Method 5: Simplifying by Using Technology
With the advent of technology, simplifying ratios has become easier. Calculators and online tools can simplify ratios quickly and accurately.
For example, let's simplify the ratio 12:18 using a calculator.
- Enter the ratio into the calculator: 12/18.
- Simplify the ratio using the calculator's simplify function.
- The simplified ratio is 2:3.

Benefits of Technology Simplification
- Quick and accurate simplification.
- Useful for ratios with large quantities.
- Saves time and effort.
In conclusion, simplifying ratios is an essential skill in mathematics and various fields. By using the five methods outlined in this article, you can simplify ratios quickly and accurately. Whether you use the greatest common divisor, cancel out common factors, convert to equivalent ratios, use proportional reasoning, or technology, simplifying ratios will become easier and more efficient.
We encourage you to practice these methods and become proficient in simplifying ratios. If you have any questions or need further clarification, please don't hesitate to comment below.
What is a ratio?
+A ratio is a way of comparing two quantities, often denoted as a fraction (e.g., 2:3 or 2/3).
Why simplify ratios?
+Simplifying ratios helps to identify equivalent ratios, makes calculations easier, and provides a clearer understanding of the relationship between quantities.
What is the greatest common divisor (GCD)?
+The greatest common divisor (GCD) is the largest number that divides both quantities without leaving a remainder.