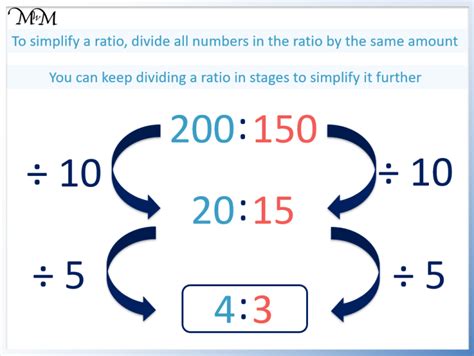
Understanding ratios is a fundamental concept in mathematics, and simplifying them to their simplest form is an essential skill that can be applied to various problems. In this article, we will explore the concept of ratios, the importance of simplifying them, and provide a step-by-step guide on how to simplify ratios to their simplest form.
The Importance of Simplifying Ratios
Ratios are used to compare two or more quantities, and they can be expressed as a fraction, proportion, or percentage. Simplifying ratios is crucial in various mathematical and real-life applications, such as cooking, finance, and science. By simplifying ratios, we can:
- Make calculations easier and more efficient
- Compare quantities more accurately
- Identify equivalent ratios and proportions
- Solve problems more effectively
Simplifying ratios to their simplest form involves reducing the fraction to its lowest terms, where the numerator and denominator have no common factors other than 1.
Understanding the Concept of Equivalent Ratios

Equivalent ratios are ratios that have the same value, but with different numbers. For example, 1:2 and 2:4 are equivalent ratios, as they represent the same proportion. To simplify ratios, we need to find the greatest common divisor (GCD) of the numerator and denominator.
What is the Greatest Common Divisor (GCD)?
The greatest common divisor (GCD) is the largest number that divides two or more numbers without leaving a remainder. To find the GCD of two numbers, we can use various methods, such as prime factorization, division, or listing the factors.
Step-by-Step Guide to Simplifying Ratios

Simplifying ratios involves the following steps:
- Identify the numerator and denominator: The numerator is the top number, and the denominator is the bottom number.
- Find the greatest common divisor (GCD): Use one of the methods mentioned earlier to find the GCD of the numerator and denominator.
- Divide both numbers by the GCD: Divide the numerator and denominator by the GCD to simplify the ratio.
Examples of Simplifying Ratios
-
Simplify the ratio 6:8 GCD of 6 and 8 is 2 Divide both numbers by 2: 6 ÷ 2 = 3, 8 ÷ 2 = 4 Simplified ratio: 3:4
-
Simplify the ratio 12:16 GCD of 12 and 16 is 4 Divide both numbers by 4: 12 ÷ 4 = 3, 16 ÷ 4 = 4 Simplified ratio: 3:4
Practical Applications of Simplifying Ratios

Simplifying ratios has various practical applications in:
- Cooking: Recipes often involve ratios of ingredients. Simplifying ratios can help reduce the amount of ingredients needed while maintaining the same flavor and texture.
- Finance: Ratios are used to compare financial data, such as income and expenses. Simplifying ratios can help identify trends and make informed decisions.
- Science: Ratios are used to describe scientific phenomena, such as the ratio of elements in a compound. Simplifying ratios can help scientists understand complex relationships.
Real-Life Examples of Simplifying Ratios
-
A recipe calls for a ratio of 2:3 of flour to sugar. If we need to make half the recipe, we can simplify the ratio by dividing both numbers by 2: 2 ÷ 2 = 1, 3 ÷ 2 = 1.5 Simplified ratio: 1:1.5
-
A company has a ratio of 4:5 of employees to managers. If the company wants to reduce the number of employees while maintaining the same ratio, they can simplify the ratio by dividing both numbers by 4: 4 ÷ 4 = 1, 5 ÷ 4 = 1.25 Simplified ratio: 1:1.25
Conclusion: Take Control of Your Ratios

Simplifying ratios is an essential skill that can be applied to various mathematical and real-life applications. By following the step-by-step guide and understanding the concept of equivalent ratios, you can simplify ratios with confidence. Remember to find the greatest common divisor (GCD) and divide both numbers by the GCD to simplify the ratio. Practice simplifying ratios with different examples, and you will become proficient in no time.
Now, it's your turn! Share your thoughts on simplifying ratios and how you apply it in your daily life. Leave a comment below and let's discuss.
What is the purpose of simplifying ratios?
+Simplifying ratios makes calculations easier and more efficient, helps compare quantities more accurately, identifies equivalent ratios and proportions, and solves problems more effectively.
How do I find the greatest common divisor (GCD) of two numbers?
+There are several methods to find the GCD of two numbers, including prime factorization, division, and listing the factors.
What are some practical applications of simplifying ratios?
+Simplifying ratios has various practical applications in cooking, finance, and science.