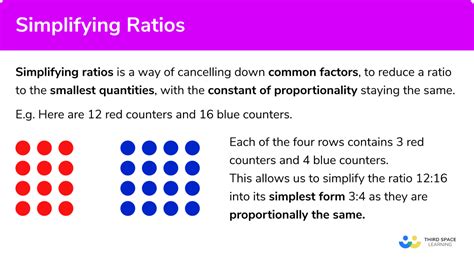
Simplifying ratios is an essential mathematical skill that can be daunting, especially when dealing with complex numbers. Fortunately, with the aid of a calculator, this task can be made significantly easier. In this article, we will explore five ways to simplify ratios using a calculator, making it a breeze to work with proportions.
Understanding Ratios and Their Importance

Ratios are used to compare quantities and are essential in various mathematical and real-world applications, such as cooking, building design, and finance. A ratio is a direct comparison of one number against another. For instance, if you have 3 apples and 4 bananas, the ratio of apples to bananas is 3:4. However, in many cases, ratios can be complex and require simplification to make them easier to understand and work with.
Method 1: Simplifying Ratios by Finding the Greatest Common Divisor (GCD)

One of the most effective methods of simplifying ratios is by finding the greatest common divisor (GCD) of the two numbers in the ratio. The GCD is the largest number that divides both numbers without leaving a remainder. To find the GCD using a calculator, follow these steps:
- Enter the two numbers in the ratio into the calculator.
- Use the calculator's GCD function (usually denoted as "GCD" or "Greatest Common Divisor") to find the GCD.
- Divide both numbers in the ratio by the GCD to simplify the ratio.
For example, if you have a ratio of 12:18, you can use the calculator to find the GCD, which is 6. Divide both numbers by 6 to simplify the ratio to 2:3.
Benefits of Using a Calculator to Find the GCD
Using a calculator to find the GCD is an efficient method of simplifying ratios, especially when dealing with large numbers. It saves time and reduces the risk of human error, making it an essential tool for anyone working with ratios.
Method 2: Simplifying Ratios by Dividing by a Common Factor

Another method of simplifying ratios is by dividing both numbers by a common factor. This method is particularly useful when the numbers in the ratio have an obvious common factor.
- Identify a common factor of both numbers in the ratio.
- Divide both numbers by the common factor to simplify the ratio.
For instance, if you have a ratio of 9:12, you can identify 3 as a common factor. Divide both numbers by 3 to simplify the ratio to 3:4.
When to Use This Method
This method is ideal when the numbers in the ratio have an obvious common factor, making it easy to simplify the ratio. However, if the numbers do not have an obvious common factor, it may be more challenging to use this method.
Method 3: Simplifying Ratios by Canceling Out Common Factors

Canceling out common factors is another method of simplifying ratios. This method involves canceling out any common factors between the two numbers in the ratio.
- Factorize both numbers in the ratio.
- Identify any common factors between the two numbers.
- Cancel out the common factors to simplify the ratio.
For example, if you have a ratio of 8:12, you can factorize both numbers as 2 x 2 x 2: 2 x 2 x 3. Cancel out the common factors (2 x 2) to simplify the ratio to 2:3.
Benefits of Canceling Out Common Factors
Canceling out common factors is an effective method of simplifying ratios, especially when the numbers in the ratio have multiple common factors. It helps to reduce the complexity of the ratio, making it easier to work with.
Method 4: Simplifying Ratios by Using a Calculator's Simplify Function

Some calculators have a built-in simplify function that can be used to simplify ratios. This function automatically simplifies the ratio to its simplest form.
- Enter the ratio into the calculator.
- Use the calculator's simplify function (usually denoted as "Simplify" or "Reduce") to simplify the ratio.
For instance, if you have a ratio of 15:20, you can use the calculator's simplify function to simplify it to 3:4.
Benefits of Using a Calculator's Simplify Function
Using a calculator's simplify function is a quick and efficient method of simplifying ratios. It saves time and reduces the risk of human error, making it an essential tool for anyone working with ratios.
Method 5: Simplifying Ratios by Finding the Lowest Terms

Finding the lowest terms is another method of simplifying ratios. This method involves finding the simplest form of the ratio by reducing it to its lowest terms.
- Enter the ratio into the calculator.
- Use the calculator to find the lowest terms of the ratio.
For example, if you have a ratio of 24:30, you can use the calculator to find the lowest terms, which is 4:5.
Benefits of Finding the Lowest Terms
Finding the lowest terms is an effective method of simplifying ratios, especially when dealing with complex numbers. It helps to reduce the complexity of the ratio, making it easier to work with.
What is a ratio?
+A ratio is a direct comparison of one number against another.
Why is it important to simplify ratios?
+Simplifying ratios is essential in various mathematical and real-world applications, such as cooking, building design, and finance.
Can I use a calculator to simplify ratios?
+Yes, you can use a calculator to simplify ratios. In fact, calculators have made it easier to work with ratios by providing various functions and methods to simplify them.
In conclusion, simplifying ratios can be made easier with the aid of a calculator. By using one of the five methods outlined in this article, you can simplify ratios with ease and accuracy. Whether you're a student, teacher, or professional, these methods will help you to work with ratios more efficiently and effectively. So, next time you're faced with a complex ratio, don't hesitate to use a calculator to simplify it.