Convert Quadratic To Vertex Form In 3 Easy Steps

Quick Links :

Quadratic equations are a fundamental concept in algebra, and understanding how to convert them to vertex form can be incredibly useful. The vertex form of a quadratic equation provides valuable insights into the parabola's shape, orientation, and key points. In this article, we will explore the importance of converting quadratics to vertex form and provide a step-by-step guide on how to do it in three easy steps.

Converting quadratics to vertex form can seem daunting at first, but it's a skill that can be mastered with practice and patience. The vertex form of a quadratic equation is particularly useful when analyzing the graph of a parabola. It allows us to identify the vertex, axis of symmetry, and the direction of the parabola's opening. Moreover, vertex form makes it easier to find the x-intercepts and the y-intercept of the parabola.

In this article, we will break down the process of converting quadratics to vertex form into three manageable steps. We will provide examples, illustrations, and practical tips to help you master this technique.

Understanding the Vertex Form

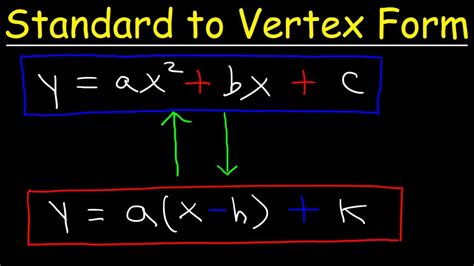
Before we dive into the steps, let's take a moment to understand the vertex form of a quadratic equation. The vertex form of a quadratic equation is written as:

y = a(x-h)^2 + k

where (h,k) represents the coordinates of the vertex. The value of 'a' determines the direction and width of the parabola, while 'h' and 'k' determine the position of the vertex.

Step 1: Identify the Coefficients

The first step in converting a quadratic to vertex form is to identify the coefficients of the quadratic equation. A quadratic equation in standard form is written as:

y = ax^2 + bx + c

We need to identify the values of 'a', 'b', and 'c' to proceed with the conversion.

For example, let's consider the quadratic equation:

y = 2x^2 + 4x + 3

In this case, the coefficients are:

a = 2 b = 4 c = 3

Step 2: Complete the Square

The second step is to complete the square. This involves manipulating the equation to express it in a perfect square trinomial form.

To complete the square, we will focus on the quadratic and linear terms. We will ignore the constant term for now.

y = 2x^2 + 4x + 3

First, we will factor out the coefficient of the quadratic term:

y = 2(x^2 + 2x) + 3

Next, we will add and subtract the square of half the coefficient of the linear term inside the parentheses:

y = 2(x^2 + 2x + 1 - 1) + 3

Now, we can rewrite the equation as:

y = 2(x+1)^2 - 2 + 3

Step 3: Write the Equation in Vertex Form

The final step is to write the equation in vertex form. We have already completed the square and expressed the equation in a perfect square trinomial form.

y = 2(x+1)^2 + 1

Comparing this with the vertex form, we can see that:

h = -1 k = 1

So, the vertex of the parabola is (-1,1).

That's it! We have successfully converted the quadratic equation to vertex form in three easy steps.

Benefits of Vertex Form

Now that we have learned how to convert quadratics to vertex form, let's explore some of the benefits of this technique.

• Easy identification of the vertex: The vertex form makes it easy to identify the coordinates of the vertex, which is a crucial point on the parabola.
• Analysis of the parabola's shape: The vertex form provides valuable insights into the parabola's shape, including its orientation and the direction of its opening.
• Finding x-intercepts and y-intercepts: The vertex form makes it easier to find the x-intercepts and y-intercept of the parabola.

Common Applications of Vertex Form

The vertex form has numerous applications in mathematics, science, and engineering. Here are some common applications:

• Graphing parabolas: The vertex form is essential for graphing parabolas, as it provides valuable insights into the parabola's shape and orientation.
• Optimization problems: The vertex form is used to solve optimization problems, such as finding the maximum or minimum value of a quadratic function.
• Physics and engineering: The vertex form is used to model real-world phenomena, such as the trajectory of a projectile or the design of a roller coaster.

Conclusion

In conclusion, converting quadratics to vertex form is a valuable technique that provides insights into the parabola's shape, orientation, and key points. By following the three easy steps outlined in this article, you can master this technique and apply it to a wide range of mathematical and real-world problems.

We hope this article has been informative and helpful. If you have any questions or comments, please feel free to share them with us.